OP Malhotra Class 12 Maths Solutions Chapter 7 Continuity and Differentiability of Functions Ex 7

Utilizing ISC S Chand Maths Class 12 Solutions Chapter 7 Continuity and Differentiability of Functions Ex 7 as a study aid can enhance exam preparation.

S Chand Class 12 ICSE Maths Solutions Chapter 7 Continuity and Differentiability of Functions Ex 7

Question 1.
Examine the continuity of the function f(x) = 2x² – 1 at x = 3.
Solution:
Given f(x) = 2x² – 1

Thus f(x) is continous at x = 3

Question 2.
Examine the following functions for continuity:
(a) f(x) = x- 5
(b) f(x) = \(\frac{1}{x-5}\), x ≠ 5
(c) f(x) = \(\frac{x^2-25}{x+5}\), x ≠ 5
(d) f(x) = |x – 5|
Solution:
(a) Given f (x) = x – 5 ; Df = R
Let c ∈ Dy = be any arbitrary point.
∴ \(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) x – 5 = c – 5 = f(c)
∴ f is continuous at x = c
but c be any arbitrary point of D_f
Thus,/is continuous at every point of its domain
∴ f be a continuous function.

(b) f(x) = \(\frac{1}{x-5}\), x ≠ 5
Here, D_f = R -{5}
Let c ∈ D_f = be any arbitrary point.

Thus, f is continuous at x = c
and c be any arbitrary point of D_f
Therefore f is continuous at every point of its domain

(c) Given \(\frac{x^2-25}{x+5}\), x ≠ 5
Here, D_f = R -{5}
Let c ∈ D_f be any arbitrary point.
∴ c ≠ – 5
Then \(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) \(\frac{x^2-25}{x-5}=\frac{c^2-25}{c-5}\)
since c ≠ – 5
∴ f(c) = \(\frac{c^2-25}{c-5}\)
∴ \(\underset{x \rightarrow c}{\mathrm{Lt}}\)f(x) = f(c)
Thus, f is continuous at x = c
but c be any arbitrary point
Hence f is continuous at every point of its domain.

(d) Given f(x) = |x – 5|
= \(\left\{\begin{array}{cc}
x-5 ; & x \geq 5 \\
-(x-5) ; & x<5
\end{array}\right.\)
D_f = R
So we examine the continuity of function of f at all x ∈ R. Let c ∈ R be any arbitrary point of D_f
There cases arises.
Case -I :
when c < 5
Then f(c) = -(c – 5)
∴ \(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) 9 (x – 5) = – (c – 5)
Thus, \(\underset{x \rightarrow c}{\mathrm{Lt}}\)f(x) = f(c)
∴ f(x) is continous for all c < 5

Case -II : when c > 5
Then f(c) = c – 5
∴ \(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) x – 5 = c – 5
Thus, \(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = f(c)
∴ f is continuous for all c > 5

Case -III :
when x = C = 5
Then f(c) = f(5) = 5 – 5 = 0

Thus f(x) is continous at x = c = 5
on combining all three cases; function f is continuous for all x ∈ R.
Thus f is continuous at every point of its domain.

Question 3.
A function f is defined by
f(x) = \(\left.\begin{array}{ll}
=\frac{x^2-4 x+3}{x^2-1}, & \text { for } x \neq 1 \\
=2, & \text { for } x=1
\end{array}\right\}\)
Test the continuity of the function at x = 1.
Solution:
Given

also f(1) = 2
∴ L.H.L = R.H.L = f(1)
Thus f is di continous at x = 1

Question 4.
Prove that the funciotn f(x) = xⁿ is continuous at x = n, where n is a positive integer.
Solution:

Question 5.
Show that the function
f(x) = \(\left\{\begin{array}{c}
x^2 \text { for } 1 \leq x<2 \\
3 x-4 \text { for } 2 \leq x<4
\end{array}\right.\)
is discontinuous at x = 2 and continuous x = 3.
Solution:

Question 6.
(a) Find all points of discontinuity of f where f is defined by
f(x) = \(\left\{\begin{array}{l}
2 x+3, \text { if } x \leq 2 \\
2 x-3, \text { if } x>2
\end{array}\right.\)
(b) Discuss the discontinuity of the function
f(x) at x = 0 , if f(x) = \(\left\{\begin{array}{l}
2 x-1, \text { if } x<0 \\
2 x+1, \text { if } x \geq 0
\end{array}\right.\)
(c) Is the function defined by
f(x) = \(\left\{\begin{array}{l}
x+5, \text { if } x \leq 1 \\
x-5, \text { if } x>1
\end{array}\right.\) a continuous funciotn?
(d) Show that
f(x) = \(\left\{\begin{array}{l}
5 x-4, \text { when } 0<x \leq 1 \\
4 x^3-3 x, \text { when } 1<x<2
\end{array}\right.\)
is continuous at x = 1.
Solution:
(a) Given f(x) = \(\left\{\begin{array}{l}
2 x+3, \text { if } x \leq 2 \\
2 x-3, \text { if } x>2
\end{array}\right.\)
Here D_f = R So we check the continous of f at all points of R. Let c ∈ D_f be any arbitrary point.
Three cases arises.
Case-I
when c < 2 then f(c) = 2c + 3
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) 2x + 3 = 2c + 3
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = f(c)
Thus f is continous for all c < 2

Case-II
when c > 2 then f(c) = 2c – 3
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) 2x – 3 = 2c – 3
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = f(c)
Hence f is continous for all c > 2

Case-III
when c = 2
\(\underset{x \rightarrow 2^{+}}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 2^{+}}{\mathrm{Lt}}\) 2x – 3 = 4 – 3 = 1
and \(\underset{x \rightarrow 2^{-}}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 2^{-}}{\mathrm{Lt}}\) 2x – 3 = 4 + 3 = 7
\(\underset{x \rightarrow 2^{-}}{\mathrm{Lt}}\) ≠ \(\underset{x \rightarrow 2^{+}}{\mathrm{Lt}}\)
Thus f is not continous i.e., discontinous at x = 2. So on contining all these cases, f is continuous at every point of its domain except x = 2.

(b) at x = 0,

Thus \(\underset{x \rightarrow 0}{\mathrm{Lt}}\) does not exists Hence f is discontinuous at x = 0

(c) Given f(x) = \(\left\{\begin{array}{l}
x+5, \text { if } x \leq 1 \\
x-5, \text { if } x>1
\end{array}\right.\)
Hence D_f = R so we examine the continuity of f at all points of R.
Let c ∈ D_f be any arbitrary point so three cases arises.
Case-I
when c < 1 then f(c) = c + 5
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) x + 5 = c + 5 = f(c)
∴ f is continuous at all c < 1

Case-II
when c > 2.
Then f(c) = c – 5
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) x – 5 = c – 5 = f(c)
∴ f is continuous at all c < 1

Case-III
when c = 1;

Thus f is discontinous at x = 1
Hence on combining all three cases, f is not continuous at every point of its domain.
∴ f is not a continuous function.

Thus f is continuous at x = 1

Question 7.
Examine the continuity for the following functions:
(a) f(x) = \(\begin{cases}x+1, & \text { if } x \geq 1 \\ x^2+1, & \text { if } x<1\end{cases}\)
(b) f(x) = \(\begin{cases}x^{10}-1, & \text { if } x \leq 1 \\ x^2, & \text { if } x>1\end{cases}\)
Solution:
(a) Given f(x) = \(\begin{cases}x+1, & \text { if } x \geq 1 \\ x^2+1, & \text { if } x<1\end{cases}\)
Here D_f = R. so we examine the continuity of f at all x ∈ R. Let c ∈ R be any real number.
Then three cases arises.
Case-I when c < 1 then f(c) = c² + 1
∴ \(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) x² + 1 = c² + 1 = f(c)
Thus f is continous at all c < 1

Case-II when c > 1 Then f(c) = c + 1
∴ \(\underset{x \rightarrow c}{\mathrm{Lt}}\) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) x + 1 = c + 1 = f(c)
∴ f is continuous for all c > 1

Case-III when c = 1;

Thus f is continuous at c = 1
So on combining all three cases, f is continuous at every point of its domain
Hence f be a continuous function.

Question 8.
Discuss the continuity of the function f(x) at x = 1/2 when f(x) is defined as follows :
f(x) = \(\left\{\begin{array}{c}
\frac{1}{2}+x, 0 \leq x<\frac{1}{2} \\
1, x=\frac{1}{2} \\
\frac{3}{2}+x, \frac{1}{2} \end{array}\right.\)
Solution:

Question 9.
Examine the continuity of the function f(x) = \(\left\{\begin{array}{c}
-2, \text { if } x \leq-1 \\
2 x, \text { if }-11
\end{array}\right.\)
Solution:
f(x) = \(\left\{\begin{array}{c}
-2, \text { if } x \leq-1 \\
2 x, \text { if }-11
\end{array}\right.\)
Here D_f = R
So we examine the continuity of f at all x ∈ R. Let c ∈ D_f be any
Case-I
when c < – 1 then f(c) = – 2
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) – 2 = – 2 = f(c)
∴ f is continuous at all c < – 1

Case-II
when – 1 < c > 1
Then f(c) = 2c
\(\underset{x \rightarrow c}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) 2x = 2c = f(c)
∴ f(x) is continuous at x = c, where – 1 < c > 1

Case-III
when c > 1
Then f(c) = 2
∴ \(\underset{x \rightarrow c}{\mathrm{Lt}}\) f (x) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\) 2 = 2 = f(c)
Thus f is continuous for all c > 1

Case-IV
when c = – 1

Thus f is continuous at x = 1
Thus on combining all five cases, f is continuous at every point of its domain.. Hence / be a continuous function.

Question 10.
A function f (x) is defined as follows: f(x) = xcos\(\frac { 1 }{ x }\), when x ≠ 0, f(0) = 0.
Examine the continuity at x = 0.
Solution:
Given f(x) = \(\begin{cases}x \cos \frac{1}{x} ; & x \neq 0 \\ 0 ; \quad x=0\end{cases}\)
Let h(x) = x ; g(x) = cos \(\frac { 1 }{ x }\)
\(\underset{x \rightarrow 0}{\mathrm{Lt}}\) h(x) = \(\underset{x \rightarrow 0}{\mathrm{Lt}}\) x = 0
Since cos\(\frac { 1 }{ x }\) is bounded in the deleted x ngd of 0 i.e., \(\left|\cos \frac{1}{x}\right|\) ≤ 1
i.e., cos\(\frac { 1 }{ x }\) is oscillating between – 1 and 1
∴ g(x) be a bounded function

∴ f is continuous at x = 0

Question 11.
The function f(x) is defined as follows:
f(x) = \(\begin{cases}(x-a) \cos \frac{1}{x-a}, & \text { when } x \neq a \\ 0, & \text { when } x=a\end{cases}\)
Examine the continuity, when x = a.
Solution:
Given

Since cos \(\frac{1}{x-a}\) is bounded function in the deleted neighbourhood of point x = a
Also, \(\left|\cos \frac{1}{x-a}\right|\) ≤ 1
i.e, cos\(\frac{1}{x-a}\) is oscilating between -1 & 1.
Thus g(x) be a bounded function.

Thus f is continuous at x = a

Question 12.
Examine the continuity of the following functions:
(a) f(x) = \(\left\{\begin{array}{r}
\frac{|x|}{x}, \text { if } x \neq 0 \\
0, \text { if } x=0
\end{array}\right.\)
(b) f(x) = \(\left\{\begin{array}{l}
\frac{|x|}{x}, \text { if } x<0 \\ -1, \text { if } x \geq 0 \end{array}\right.\)
Solution:
(a)

So we examine the continuity of f at all x ∈ R.
Let c ∈ R be any real number
Case-I when c > 0 Then f(c) = 1

Thus f is discontinuous at x = 1
Therefore on combining all three cases, f is continuous at every point of its domain except at x = 0

(b) Given

Thus f is continuous at x = 0

Question 13.
Examine the continuity at x = 0
(a) f(x) = \(\left\{\begin{array}{c}
\frac{\tan 2 x}{3 x}, \text { when } x \neq 0 \\
\frac{2}{3}, \text { when } x=0
\end{array}\right.\)
(b) f(x) = 1 + \(\frac{|x|}{x}\) for x ≠ 0 and f(0) = 1.
Solution:

Question 14.
Find the value of value of a, if the function f (x) defined by
f(x) = \(\left\{\begin{array}{r}
2 x-1, x<2 \\ a, x=2 \\ x+1, x>2
\end{array}\right.\)
is continuous at x = 2.
Solution:

Question 15.
Find the relationship between a and 6 so that the function defined by f(x) = \(\left\{\begin{array}{l}
a x+1, \text { if } x \leq 3 \\
b x+3, \text { if } x>3
\end{array}\right.\) is continuous at x = 3.
Solution:
Given

which is the required relation between a & b.

Question 16.
For what value of X is the function f(x) = \(\begin{cases}\lambda\left(x^2-2 x\right), & \text { if } x \leq 0 \\ 4 x+1, & \text { if } x>0\end{cases}\) continous at x = 0?
Solution:
at x = 0

∴ L.H.Limit ≠ R.H.Limit
Thus f is not continuous at x = 0 i.e.
at any real value of λ.
at x = 1 :
\(\underset{x \rightarrow 1}{\mathrm{Lt}}\)f(x)= \(\underset{x \rightarrow 1}{\mathrm{Lt}}\)4x+ 1 = 4 + 1 =5 = f(1)
∴ f is continuous at x = 1

Question 17.
Find the value of k, so that the function f defined by
f(x) = \(\begin{cases}k x+1, & \text { if } x \leq \pi \\ \cos x, & \text { if } x>\pi\end{cases}\)
is continuous at x = π.
Solution:

Question 18.
Find the value of k, for which
f(x) = \(\begin{cases}\frac{\sqrt{1+k x}-\sqrt{1-k x}}{x} & \text { if }-1 \leq x<0 \\ \frac{2 x+1}{x-1}, & \text { if } 0 \leq x<1\end{cases}\)
is continuous at x = 0.
Solution:
at x = 0

Question 19.
If the following fimctoin f(x) is continuous at x = 0, then find the value of k.
f(x) = \(\left\{\begin{array}{cc}
\frac{1-\cos 2 x}{2 x^2}, & x \neq 0 \\
k, & x=0
\end{array}\right.\)
Solution:

Question 20.
Prove that the funciton
f(x) = \(\left\{\begin{array}{cc}
\frac{x}{|x|+2 x^2}, & x \neq 0 \\
k, & x=0
\end{array}\right.\)
remains discontinuous at x = 0, regardless of the choice of k.
Solution:

Thus f is remains discontinuous at x = 0 regardless of the choice of k i.e. whatever the real value of k may be. On removable discontinuity;

Question 21.
The function f(x) = \(\frac{2 x^2-8}{x-2}\) is undefined at x = 2. What value should be assigned to f (2) so that f (x) is continuous at x = 2 ?
Solution:

Since f(x) is continuous at x = 2
if \(\underset{x \rightarrow 2}{\mathrm{Lt}}\)f(x) = f(2) if 8 = f(2)
Thus required value of f(2) which is assigned be 8.

Question 22.
The function f(x) = \(\frac{x^2-1}{x^3-1}\) at the point x = 1 ; what should be the value of f (1) such that f (x) may be continuous at x = 1?
Solution:

Now f(x) may be continuous at x = 1
if \(\underset{x \rightarrow 1}{\mathrm{Lt}}\)f(x) = f(1) if \(\frac { 2 }{ 3 }\) = f(1)

Question 23.
Is the function f(x) = \(\frac{3 x+2 \sin x}{x}\) continuous at x = 0 ? If not, how may the function be defined at x = 0 to make it continuous at that point ?
Solution:
Given f(x) = \(\frac{3 x+2 \sin x}{x}\)
Since f(o) be indeterminate form i.e. (\(\frac { 0 }{ 0 }\)) form.
Thus f(x) is not defined at x = 0
∴ f(x) is not continuous at x = 0

∴ f(x) is continuous at x = 0.

On differentiability

Question 24.
Show that the function f(x)=|x – 3|, x ∈ R, is continuous at x = 3 but not differentiable x = 3.
Solution:
Given

∴ f is continuous at x = 3
∴ f is continuous at x = 30
Differentiability at x = 3

Thus, f is not differentiable x = 3
Hence f is continuous but not differentiable at x = 3

Question 25.
Show that the function
f(x) = |x-1| + |x + 1| for all x ∈ R, is not differentiable at the points x = – 1 and x = 1.
Solution:
f(x) = |x-1| + |x + 1|

Question 26.
Show that the function
f(x) = \(\begin{cases}x-1, & \text { if } x<2 \\ 2 x-3, & \text { if } x \geq 2\end{cases}\)
is not differentiable at x = 2.
Solution:

Question 27.
Show that f(x) = | x – 20| is continuous at x = 20 but f'(x) does not exist at x = 20.
Solution:

Examples

Question 1.
A real valued function f is continuous at a point x = a if it is defined at x = a and iff \(\lim _{x \rightarrow a^{-}}\) f(x) = \(\lim _{x \rightarrow a^{+}}\) f(x)
Solution:
f(a) [∵ value of limit = value of function at the same point]

Question 2.
A real function f (x) is said to be differentiable at x = a, if …………….
Solution:
Rf ‘ (a) = Lf ‘ (a)

Question 3.
If u and v are two function of x, then derivative of \(\frac { u }{ v }\) i.e., (\(\frac { u }{ v }\))’ = ……………..
Solution:
\(\left(\frac{u}{v}\right)^{\prime}=\frac{v u^{\prime}-u v^{\prime}}{v^2}\) ; v ≠ 0

Question 4.
If the graph of a function/can be drawn around a point without lifting the pen from the paper, then the function is ……………..
Solution:
Continuous

Question 5.
If the graph of a function around a point cannot be drawn without lifting the pen from the paper, then function is ……………..
Solution:
Discontinuous

Question 6.
Examine the continuity of the function.
f(x) = \(\left.\begin{array}{c}
x^2 \text { when } x \neq 1 \\
2 \text { when } x=1
\end{array}\right\}\) at x = 1.
The function is ………………. at x = 1.
Solution:
\(\underset{x \rightarrow 1}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 1}{\mathrm{Lt}}\)x² = 1² = 1
and f(1) = 2
∴ \(\underset{x \rightarrow 1}{\mathrm{Lt}}\) f(x) ≠ f(1)
∴ The function is discontinuous at x = 1.

Question 7.
The graph of a function has a …………….. as shown in fig. and so it is a …………….. function.

Solution:
Jump, discontinuous

Question 8.
The graph of a function has a …………….. as shown in fig. and so it is a …………….. function.
Solution:
hole, discontinuous

Question 9.
The function f(x) = x³ – 7x² + 5 being a …………….. function is ……………..
Solution:
polynomial, continuous Tick the correct answer.

Question 10.
The composition gof of two continuous functions is continuous/discontinuous.
Solution:
continuous

Question 11.
f(x) = \(\left\{\begin{array}{c}
3 x,-8, \text { if } x \leq 5 \\
2 k, \text { if } x>5
\end{array}\right.\) is continuous, find k
(a) \(\frac { 3 }{ 7 }\)
(b) \(\frac { 7 }{ 2 }\)
(c) \(\frac { 2 }{ 7 }\)
(d) \(\frac { 4 }{ 7 }\)
Solution:

Question 12.
If f(x) = 2x and g (x) = \(\frac { x² }{ 2 }\) + 1, then which of the following can be a discontinuous function
(a) f(x) + g(x)
(b) f(x) – g(x)
(c) f(x).g(x)
(d) \(\frac{g(x)}{f(x)}\)
Solution:
Given f(x) = 2x; g(x) = \(\frac { x² }{ 2 }\) + 1
For option (A); f(x) + g(x) = 2x + \(\frac { x² }{ 2 }\) – 1,
which is polynomial function and hence continuous everywhere.
For option (B); f(x) – g(x) = 2x – \(\frac { x² }{ 2 }\) – 1,
which is polynomial function in x and hence continuous everywhere.
For option (C); f(x) g(x) = 2x (\(\frac { x² }{ 2 }\) + 1) = x³ + 2x.
which is polynomial in x and hence continuous everywhere.
In option (D); \(\frac{g(x)}{f(x)}=\frac{\frac{x^2}{2}+1}{2 x}=\frac{x^2+2}{4 x}\)
which does not exists at x = 0 and hence discontinuous at x = 0.

Question 13.
f(x) = \(\left\{\begin{array}{c}
3 x,-8, \text { if } x \leq 5 \\
2 k, \text { if } x>5
\end{array}\right.\) is continuous, find k.
(a) \(\frac { 3 }{ 7 }\)
(b) \(\frac { 7 }{ 2 }\)
(c) \(\frac { 2 }{ 7 }\)
(d) \(\frac { 4 }{ 7 }\)
Solution:

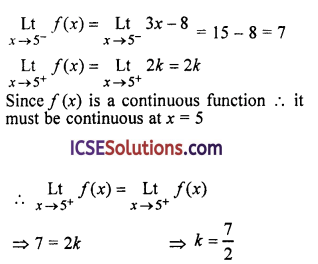
Question 14.
The function f (x) = \(\frac{4-x^2}{4 x-x^3}\)
(a) discontinuous at only one point
(b) discontinuous exactly at two points
(c) discontinuous exactly at three points
(d) none of these.
Solution:
Given f(x) = \(\frac{4-x^2}{4 x-x^3}\)
since f(x) is not defined at 4x – x³ = 0
i.e. x(4 – x²) = 0
⇒ x = 0, ±2
Thus f(x) is discontinuous exactly at three points x = 0, ± 2.
Since we know that, f (x) is continuous at x = a, Then f(x) should be defined at x = a
and \(\underset{x \rightarrow a}{\mathrm{Lt}}\)f(x) = f(a)

Question 15.
Let f and g be two real functions at a real number c. Then, which of the following statements is/are true?
I. f+ g is a continuous at x = c.
II. f- g is continuous at x = c.
III. f.g is continuous at x = c.
IV. \(\frac { f }{ g }\) is continuous at x = 0 provided g (x) ≠ 0
(a) I and II
(b) II and III
(c) I, II and III
(d) All
Solution:
by standard results.

Question 16.
A real function f is said to be continuous, if it is continuous at every point in the
(a) domain of f
(b) co-domain of f
(c) range off
(d) none of these
Solution:
(a) domain of f

Question 17.
If f(x) = \(\left\{\begin{array}{rll}
k x^2, & \text { if } & x \leq 2 \\
3, & \text { if } & x>2
\end{array}\right.\)
is continuous at x = 2,
then the value of k is
(a) \(\frac { 4 }{ 3 }\)
(b) – \(\frac { 4 }{ 3 }\)
(c) 3
(d) 4
Solution:

Question 18.
Which of the following functions is/are continuous?
I. constant function
II. polynomial function
III. modulus function
IV. sine function
(a) only I
(b) only II
(c) II, III and IV
(d) All of these
Solution:
(d) All of these

Question 19.
The function f (x) = 2x² + cos x + e^x – 2 is
(a) discontinuous at x = 0
(b) discontinuous at x = π
(c) discontinuous at x = \(\frac { π }{ 2 }\)
(d) continuous at all points
Solution:
Since polynomial, constant, exponential functions are continuous everywhere. Further, cosine function is continuous function.
Also, sum of continuous functions is continuous.
Thus the given function f (x) = 2x² + cos x + e^x – 2
is continuous at all points.

Question 20.
If the function f(x) = \(\left\{\begin{array}{ccc}
\frac{x^2-1}{x-1}, & \text { when } & x \neq 1 \\
k, & \text { when } & x=1
\end{array}\right.\)
is given to be continuous at x = 1, then the value of k is
(a) – 2
(b) 3
(c) \(\frac { 1 }{ 2 }\)
(d) 2
Solution:
Let \(\underset{x \rightarrow 1}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 1}{\mathrm{Lt}}\)\(\frac{x^2-1}{x-1}\)
= \(\underset{x \rightarrow 1}{\mathrm{Lt}}\)\(\frac{(x+1)(x-1)}{(x-1)}\) = \(\underset{x \rightarrow c}{\mathrm{Lt}}\)x +1 = 1 + 1 = 2
and f(1) = k
Since f(x) is continuous at x = 1
∴ \(\underset{x \rightarrow 1}{\mathrm{Lt}}\)f(x) = f(1) ⇒ 2 = k

Question 21.
The number of points of discontinuity of/ defined by | x | – | x + | is
(a) 1
(b) 2
(c) 0
(d) None of these
Solution:
f(x) = | x | – | x + 1 |

Thus, f(x) is continuous at x = 0.
Further polynomial function and modulus function are all continuous and difference of two continuous function is continuous. Thus f (x) is continuous everywhere.
∴ The number of points of discontinuity of f be 0.

Question 22.
If a function f is differentiable at c, then it is
(a) discontinuous at c
(b) continuous at c
(c) not defined at c
(d) None of these
Solution:
Given f(x) is differentiable at x = c

Question 23.
Which of the following is not a correct statement?
A function is not differentiable at x = a, if
(a) either or both Rf’ (a) and Lf’ (a) do not exist
(b) both Rf’ (a) and Lf’ exist (a) exist but are not equal
(c) Rf’ (a) and Lf’ (a) both exist and are equal
(d) either or both Rf’ (a) and Lf’ (a) are not finite
Solution:
(c) Rf’ (a) and Lf’ (a) both exist and are equal
[if Lf’ (a) = Rf’ (a) Then f (x) is diff. at x = a]

Question 24.
Let f(x) = \(\left\{\begin{array}{cll}
c x^2+2 x, & \text { if } & x<2 \\
2 x+4, & \text { if } & x \geq 1
\end{array}\right.\). If the function is continuous on (- ∞, ∞), then the value of a is equal to
(a) 4
(b) 2
(c) 3
(d) 1
Solution:

Question 25.
The function f(x) = \(\left\{\begin{array}{cl}
x-1, & x<2 \\
2 x-3, & x \geq 2
\end{array}\right.\) is
continuous function
(a) at x = 2 only
(b) for all integral values of x only
(c) for all real values of x
(d) for all real values of x such that x ≠ 2
Solution:
When x < 2 ; f (x) = x – 1 which is a polynomial function and hence continuous everywhere.
When x > 2 ; f(x) = 2x – 3, which is a polynomial function and hence continuous everywhere, at x = 2

= 4 – 3 = 1 and f (2) = 1
∴ f(x) is continuous at x = 2
Thus f (x) is continuous for all real values of x.

Question 26.
Let f (x) be a function differentiable at x = c, then \(\lim _{x \rightarrow c}\) f(x) equals
(a) f(c)
(b) f ”(x)
(c) \(\frac { 1 }{ f(c) }\)
(d) None of these
Solution:
Given f(x) is differentiable at x = c

Question 27.
If f(x) = \(\left\{\begin{array}{cl}
\frac{1-\cos p x}{x \sin x}, & x \neq 0 \\
\frac{1}{2}, & x=0
\end{array}\right.\)
is continuous at x = 0, then p is equal to
(a) 2
(b) – 2
(c) 1, – 1
(d) none of these
Solution:

Question 28.
The function f (x) = | sin x |
(a) f is differentiable everywhere
(b) f is continuous everywhere but not differentiable at x = nπ, n ∈ Z
(c) f is continuous everywhere but not differentiable at x = (2n + 1) \(\frac { π }{ 2 }\), n ∈ Z
(d) none of these
Solution:
f(x) = | sin x | = \(\left\{\begin{aligned}
-\sin x ; & x \sin x ; & x \geq n \pi
\end{aligned}\right.\)
n = even
at x = nπ (n even)

Thus f(x) is not differentiable at x = nπ (n = odd)
Hence f(x) is not differentiable at x = nπ, n ∈ Z.

Question 29.
If f(x) = \(\left\{\begin{array}{cc}
m x+1, & x \geq \frac{\pi}{2} \\
\sin x+n, & x<\frac{\pi}{2}
\end{array}\right.\) is continuous at x = \(\frac { π }{ 2 }\), then
(a) m = 1, n = 0
(b) m = \(\frac { nπ }{ 2 }\) + 1
(c) n = \(\frac { mπ }{ 2 }\)
(d) m = n = \(\frac { π }{ 2 }\)
Solution:

Question 30.
Determine the value of constant ‘k’ so that the function f (x) = \(\left\{\begin{array}{r}
\frac{k x}{|x|}, x<0 \\
3, x \geq 0
\end{array}\right.\) is continuous at x = 0.
Solution:
At x = 0
L.H.L = \(\underset{x \rightarrow 0^{-}}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 0^{-}}{\mathrm{Lt}}\) \(\frac{k x}{-x}\) = – k
R.H.L = \(\underset{x \rightarrow 0^{+}}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 0}{\mathrm{Lt}}\) 3 = 3
and f(0) = 3
Since f(x) is continuous at x = 0.
∴ L.H.L = R.H.L = f(0)
Thus, – k = 3 ⇒ k = – 3

Question 31.
If the function f defined as f(x) = \(\left\{\begin{array}{cc}
\frac{x^2-9}{x-3}, & x \neq 3 \\
k & x=3
\end{array}\right.\)
is continuous at x = 3, find the value of A.
Solution:

Question 32.
If f(x) = \(\left\{\begin{array}{cc}
x^2, & \text { when } x \neq 1 \\
0 & \text { when } x=1
\end{array}\right.\) find whether it is continuous or discontinuous at x = 1.
Solution:
\(\underset{x \rightarrow 1}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 1}{\mathrm{Lt}}\) x² = 1 and f (1) = 0
∴ \(\underset{x \rightarrow 1}{\mathrm{Lt}}\) f(x) ≠ f(1)
Thus, f is not continuous at x = 0.

Question 33.
If the function
f(x) = \(\left\{\begin{array}{cc}
\frac{\sin \frac{3 x}{2}}{x}, & x \neq 0 \\
k, & 0
\end{array}\right.\) is continuous
at x = 0, then write the value of A.
Solution:

Question 34.
Examine the continuity of the function f(x) = x³ + 2x² – 1.
Solution:
Given f (x) = x³ + 2x² – 1
Clearly f (x) being a polynomial function and hence continuous at every real point x ∈ R.

Question 35.
Examine the continuity of
f(x) = \(\left\{\begin{array}{rll}
3 x+5, & \text { if } & x \geq 2 \\
x^2, & \text { if } & x<2 \end{array}\right.\)
Solution:
Case-I: When x > 2 ; f(x) = 3x + 5
We know that, every polynomial function is continuous everywhere.
∴ f(x) is continuous at each x > 2.

Case-II: When x < 2 ; f{x) = x², which is polynomial in x and hence continuous everywhere.
Thus, f(x) is continuous at each x < 2.

Case-III : at x = 2
\(\underset{x \rightarrow 2^{+}}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 2^{+}}{\mathrm{Lt}}\) 3x + 5 = 6 + 5 = 11
\(\underset{x \rightarrow 2^{-}}{\mathrm{Lt}}\) f(x) = \(\underset{x \rightarrow 2^{-}}{\mathrm{Lt}}\) x² = 4
∴ \(\underset{x \rightarrow 2^{+}}{\mathrm{Lt}}\) f(x) ≠ \(\underset{x \rightarrow 2^{-}}{\mathrm{Lt}}\) f(x)
Thus f (x) is discontinuous at x = 2
Hence f (x) is continuous everywhere except at x = 2.

Question 36.
Examine the continuity of
f(x) = \(\left\{\begin{array}{cl}
\frac{|x-4|}{2(x-4)}, & \text { if } x \neq 4 \\
0, & \text { if } x=4
\end{array} \text { at } x=4\right.\)
Solution:

Question 37.
Examine the continuity
f(x) = |x| + |x – 1|, at x = 1
Solution:
Given f(x) = | x | + | x – 1 |

Thus, f(x) is continuous at x = 1.

Question 38.
If f(x) = 2 | x | + 3 | sin x | + 6, then the right hand derivative off (x) at x = 0 is ……………
Solution:

Question 39.
Let f(x) = x | x |, for all x ∈ R, check its differentiability at x = 0.
Solution: