ML Aggarwal Class 12 Maths Solutions Section B Chapter 2 Three Dimensional Geometry Ex 2.3

The availability of ML Aggarwal Class 12 ISC Solutions Chapter 2 Three Dimensional Geometry Ex 2.3 encourages students to tackle difficult exercises.

ML Aggarwal Class 12 Maths Solutions Section B Chapter 2 Three Dimensional Geometry Ex 2.3

Very short answer type questions (1 to 5):

Question 1.
Find the angle between each of the following pairs of lines:

(i) \(\vec{r}=4 \hat{i}-\hat{j}+\lambda(\hat{i}+2 \hat{j}-2 \hat{k})\)
and \(\vec{r}=\hat{i}-\hat{j}+2 \hat{k}-\mu(2 \hat{i}+4 \hat{j}-4 \hat{k})\)

(ii) \(\vec{r}=3 \hat{i}-2 \hat{j}+6 \hat{k}+\lambda(2 \hat{i}+\hat{j}+2 \hat{k})\)
and \(\vec{r}=2 \hat{j}-5 \hat{k}+\mu(6 \hat{i}+3 \hat{j}+2 \hat{k})\) (NCERT Exemplar)

(iii) \(\vec{r}=3 \hat{i}+\hat{j}-2 \hat{k}+\lambda(\hat{i}-\hat{j}-2 \hat{k})\)
and \(\vec{r}=2 \hat{i}-\hat{j}-5 \hat{k}+\mu(3 \hat{i}-5 \hat{j}-4 \hat{k})\)
Solution:
(i) Given eqns. of lines are
\(\vec{r}=(4 \hat{i}-\hat{j})+\lambda(\hat{i}+2 \hat{j}-2 \hat{k})\) ………………….(1)
\(\vec{r}=\hat{i}-\hat{j}+2 \hat{k}-\mu(2 \hat{i}+4 \hat{j}-4 \hat{k})\) ……………………(2)
Let \(\overrightarrow{m_1}\) and \(\overrightarrow{m_2}\) vectors parallel to line (1) and (2)
∴ \(\overrightarrow{m_1}=\hat{i}+2 \hat{j}-2 \hat{k}\) ;
\(\overrightarrow{m_2}=2 \hat{i}+4 \hat{j}-4 \hat{k}\)
Then cos θ = \(\frac{\overrightarrow{m_1} \cdot \overrightarrow{m_2}}{\left|\overrightarrow{m_1}\right|\left|\overrightarrow{m_2}\right|}\)
= \(\frac{(\hat{i}+2 \hat{j}-2 \hat{k})(2 \hat{i}+4 \hat{j}-4 \hat{k})}{\sqrt{1+4+4} \sqrt{4+16+16}}\)
cos θ = \(\frac{1(2)+2(4)-2(-4)}{3 \times 6}\)
= \(\frac{18}{18}\) = 1
Thus θ = 0°

(ii) Given lines parallel to the vectors
\(\vec{b}=2 \hat{i}+\hat{j}+2 \hat{k}\)
and \(\vec{c}=6 \hat{i}+3 \hat{j}+2 \hat{k}\)
Let θ be the acute angle between two given lines
Then cos θ = \(\frac{|\vec{b} \cdot \vec{c}|}{|\vec{b}||\vec{c}|}\) ………………..(1)
\(\vec{b} \cdot \vec{c}=(2 \hat{i}+\hat{j}+2 \hat{k}) \cdot(6 \hat{i}+3 \hat{j}+2 \hat{k})\)
= 2 (6) + 1 (3) + 2 (2) = 19
and \(|\vec{b}|=\sqrt{2^2+1^2+2^2}\) = 3
\(|\vec{c}|=\sqrt{6^2+3^2+2^2}\) = 7
∴ from (1) ;
cos θ = \(\frac{19}{3 \times 7}=\frac{19}{21}\)
θ = cos^-1 \(\left(\frac{19}{21}\right)\)

(iii) Given pair of lines are

Question 2.
Find the angle between each of the following pairs of lines :
(i) \(\frac{x+3}{3}=\frac{y-1}{5}=\frac{z+3}{4}\) and \(\frac{x+1}{1}=\frac{y-4}{1}=\frac{z-5}{2}\) (NCERT)
(ii) \(\frac{x+1}{2}=\frac{y-2}{5}=\frac{z+3}{4}\) and \(\frac{x-1}{5}=\frac{y+2}{2}=\frac{z-1}{-5}\) (ISC 2020)
(iii) \(\frac{x}{2}=\frac{y}{2}=\frac{z}{1}\) and \(\frac{x-5}{4}=\frac{y-2}{1}=\frac{z-3}{8}\) (NCERT)
Solution:
(i) The D’ratios of given lines are
< 3, 5, 4 > and < 1, 1, 2 >
If θ be the required angle between them
cos θ = \(\frac{3(1)+5(1)+4(2)}{\sqrt{9+25+16} \sqrt{1^2+1^2+2^2}}\)
= \(\frac{16}{10 \sqrt{3}}\)
= \(\frac{8}{5 \sqrt{3}}\)
⇒ θ = cos^-1 (\(\frac{8}{5 \sqrt{3}}\))

(ii) eqn. of given lines are
\(\frac{x+1}{2}=\frac{y-2}{5}=\frac{z+3}{4}\) ………………(1)
\(\frac{x-1}{5}=\frac{y+2}{2}=\frac{z-1}{-5}\) ……………….(2)
The direction ratios of line (1) and (2) are ;
< 2, 5, 4 > and < 5, 2, – 5 >
Let θ be the acute angles between lines (1) and (2) be given by
cos θ = \(\frac{|2 \times 5+5 \times 2+4(-5)|}{\sqrt{2^2+5^2+4^2} \sqrt{5^2+2^2+(-5)^2}}\)
= 0
⇒ θ = \(\frac{\pi}{2}\)

(iii) The Direction ratios of given two lines are
< 2, 2, 1 > and < 4, 1, 8 >
If θ be the angle between them
Then cos θ = \(\frac{|2 \cdot 4+2 \cdot 1+1 \cdot 8|}{\sqrt{4+4+1} \sqrt{16+1+64}}\)
cos θ = \(\frac{18}{3 \times 9}\)
= \(\frac{18}{27}=\frac{2}{3}\)
⇒ θ = cos^-1 \(\frac{2}{3}\)

Question 2 (old).
(ii) \(\frac{x-2}{2}=\frac{y-1}{5}=\frac{z+3}{-3}\) and \(\frac{x+1}{-1}=\frac{y-4}{8}=\frac{z-5}{4}\) (NCERT)
Solution:
The D’s ratios of given lines are < 2, 5, – 3 > and < – 1, 8, 4 >
If θ be the required angle between them
cos θ = \(\frac{-2+40-12}{\sqrt{4+25+9} \sqrt{1+64+16}}\)
= \(\frac{26}{\sqrt{38} \times 9}\)
⇒ θ = cos^-1 (\(\frac{26}{9 \sqrt{38}}\))

Question 3.
Show that the lines \(\frac{x-5}{7}=\frac{2-y}{5}=\frac{z}{1}\) and \(\frac{3-x}{-1}=\frac{y}{2}=\frac{z+1}{3}\) are at right angles.
Solution:
Given lines can be written as ;
\(\frac{x-5}{7}=\frac{y-2}{-5}=\frac{z}{1}\) ……………………..(1)
and \(\frac{x-3}{1}=\frac{y}{2}=\frac{z+1}{3}\) ……………….(2)
On comparing with
\(\frac{x-x_1}{a_1}=\frac{y-y_1}{b_1}=\frac{z-z_1}{c_1}\)
and \(\frac{x-x_2}{a_2}=\frac{y-y_2}{b_2}=\frac{z-z_2}{c_2}\)
a₁ = 7 ;
b₁ = – 5 ;
c₁ = 1

a₂ = 1 ;
b₂ = 2 ;
c₂ = 3
Both lines are at right angles
iff a₁a₂ + b₁b₂ + c₁c₂ = 0
Here a₁a₂ + b₁b₂ + c₁c₂ = 7 (1) – 5 (2) + 1 (3) = 0
∴ given lines (1) and (2) are perpendicular.

Question 3 (old).
(i) Show that the lines \(\frac{x-5}{7}=\frac{y+2}{-5}=\frac{z}{1}\) and \(\frac{x}{1}=\frac{y}{2}=\frac{z}{3}\) are at right angles. (NCERT)
Solution:
The eqns. of lines are
\(\frac{x-5}{7}=\frac{y+2}{-5}=\frac{z}{1}\) ……………….(1)
and \(\frac{x}{1}=\frac{y}{2}=\frac{z}{3}\) ………………..(2)
The Direction ratios of lines (1) and (2) are < 7, – 5, 1 > and < 1, 2, 3 >.
[We know that the lines having D’ratios < a₁, b₁, c₁ > and < a₂, b₂, c₂ > are ⊥
iff a₁a₂ + b₁b₂ + c₁c₂ = 0]
Here a₁a₂ + b₁b₂ + c₁c₂
= 7 (1) + (- 5) 2 + 1 (3)
= 7 – 10 + 3 = 0
line (1) and line (2) are mutually perpendicular.

Question 4.
(i) If the lines \(\frac{x-1}{-3}=\frac{y-2}{2 k}=\frac{z-3}{2}\) and \(\frac{x-1}{3 k}=\frac{y-1}{1}=\frac{z-6}{-5}\) are perpendicular, then find the value of k.
(ii) FDinds the value of k so that the lines x = – y = kz and x – 2 = 2y + 1 = – z + 1 are perpendicular to each other.
Solution:
(i) Given eqns. of lines are
\(\frac{x-1}{-3}=\frac{y-2}{2 k}=\frac{z-3}{2}\) ………………….(1)
and \(\frac{x-1}{3 k}=\frac{y-1}{1}=\frac{z-6}{-5}\) ………………..(2)
Here a₁ = – 3 ;
b₁ = 2λ ;
c₁ = 2 ;
a₂ = 3λ ;
b₂= 1
c₂ = – 5
Now lines (1) and (2) are perpendicular.
∴ a₁a₂ + b₁b₂ + c₁c₂ = 0
– 3 (3λ) + 2λ (1) + 2 (- 5) = 0
– 7λ – 10 = 0
⇒ λ = – \(\frac{10}{7}\)

(ii) eqns. of given lines van be written as ;
\(\frac{x-2}{1}=2\left(y+\frac{1}{2}\right)\) …………………..(1)
and \(\frac{x-2}{1}=2\left(y+\frac{1}{2}\right)\) = – (z – 1)
⇒ \(\frac{x-2}{2}=\frac{y+\frac{1}{2}}{1}=\frac{z-1}{-2}\) …………………(2)
The direction ratios of lines (1) and (2) are < k, – k, 1 > and < 2, 1, – 2 >
Since the lines (1) and (2) are ⊥ to each other
∴ cos θ = 0
⇒ 0 = (k) (2) – k × 1 + 1 × (- 2)
[∵ a₁a₂ + b₁b₂ + c₁c₂ = 0]
⇒ 2k – k – 2 = 0
⇒ k = 2

(iii) Given lines can be written as
\(\frac{x-5}{5 \lambda+2}=\frac{+y-2}{-5}=\frac{z-1}{1}\) ………………(1)
and \(\frac{x}{1}=\frac{y+1 / 2}{2 \lambda}=\frac{z-1}{3}\) ………………(2)
Direction ratios of line (1) are proportional
to,< 5λ + 2, – 5, 1 >
i.e. a₁ = 5 + 2 ;
b₁ = – 5 ;
c₁ = 1
and D’ratios of line (2) be proportional to < 1, 2λ, 3 >
Here a₂ = 1 ;
b₂ = 2λ
c₂ = 3
lines (1) and (2) are perpendicular to each other
∴ a₁a₂ + b₁b₂ + c₁c₂ = 0
⇒ (5λ + 2) . 1 – 5 (2λ) + 1 (3) = 0
⇒ 5λ + 2 -10λ + 3 = 0
⇒ – 5λ + 5 = 0
⇒ λ = 1.

Question 4 (old).
(ii) Find the values of λ so that the lines \(\frac{1-x}{3}=\frac{y-2}{2 \lambda}=\frac{z-3}{2}\) and \(\frac{x-1}{3 \lambda}=\frac{y-1}{1}=\frac{6-z}{7}\) are perpendicular to each other.
(iii) Find the values of λ so that the following lines are perpendicular to each other :
\(\frac{x-5}{5 \lambda+2}=\frac{2-y}{5}=\frac{1-z}{-1}\) ; \(\frac{x}{1}=\frac{2 y+1}{4 \lambda}=\frac{1-z}{-3}\)
Solution:
(ii) Given lines can be written as
\(\frac{x-1}{-3}=\frac{y-2}{2 \lambda}=\frac{z-3}{2}\) ………………..(1)
\(\frac{x-1}{3 \lambda}=\frac{y-1}{1}=\frac{z-6}{-7}\) ………………..(2)
Here a₁ = – 3 ;
b₁ = 2λ
c₁ = 2
a₂ = 3λ
b₂ = 1
c₂ = – 7
Two given lines (1) and (2) are perpendicular
∴ a₁a₂ + b₁b₂ + c₁c₂ = 0
– 3 (3λ) + 2λ (1) + 2 (- 7) = 0
⇒ – 7λ – 14 = 0
⇒ λ = – 2.

Question 5.
Find the angle between each of the following pairs of lines :
(i) \(\frac{x-2}{3}=\frac{y+1}{-2}\), z = 2 and \(\frac{x-1}{1}=\frac{y+3}{3}=\frac{z+5}{2}\)
(ii) \(\frac{5-x}{3}=\frac{y+3}{-2}\), z = 5 and \(\frac{x-1}{1}=\frac{1-y}{3}=\frac{z-5}{2}\)
(iii) \(\frac{x}{1}=\frac{z}{-1}\), y = 0 and \(\frac{x}{3}=\frac{y}{4}=\frac{z}{5}\)
Solution:
(i) Given lines can be written as;
\(\frac{x-2}{3}=\frac{y+1}{-2}=\frac{z-2}{0}\) …………………….(1)
and \(\frac{x-1}{1}=\frac{y+3}{3}=\frac{z+5}{2}\) ………………….(2)
∴ Direction ratios of two lines (1) and (2) are given by < 3, – 2, 0 > and < 1, 3, 2 >
Thus, a₁ = 3,
b₁ = – 2 ;
c₁ = 0 ;
a₂ = 1;
b₂ = 3 ;
c₂ = 2
Let θ be the acute angle between given lines
Then cos θ = \(\frac{\left|a_1 a_2+b_1 b_2+c_1 c_2\right|}{\sqrt{a_1^2+b_1^2+c_1^2} \sqrt{a_2^2+b_2^2+c_2^2}}\)
= \(\frac{|3(1)-2(3)+0(2)|}{\sqrt{3^2+(-2)^2+0^2} \sqrt{1^2+3^2+2^2}}\)
⇒ cos θ = \(\frac{3}{\sqrt{13} \sqrt{14}}=\frac{3}{\sqrt{182}}\)
θ = cos^-1 \(\frac{3}{\sqrt{182}}\)

(ii) Given lines can be written as :
\(\frac{x-5}{-3}=\frac{y+3}{-2}=\frac{z-5}{0}\) ………………….(1)
and \(\frac{x-1}{1}=\frac{y-1}{-3}=\frac{z-5}{2}\) …………………..(2)
∴ Direction ratios of two lines (1) and (2) are given by
< – 3, – 2, 0 > and < 1, – 3, 2 >
Here a₁ = – 3 ;
b₁ = – 2 ;
c₁ = 0 ;
a₂ = 1 ;
b₂ = – 3 ;
c₂ = 2
Then cos θ = \(\frac{\left|a_1 a_2+b_1 b_2+c_1 c_2\right|}{\sqrt{a_1^2+b_1^2+c_1^2} \sqrt{a_2^2+b_2^2+c_2^2}}\)
= \(\frac{\left|a_1 a_2+b_1 b_2+c_1 c_2\right|}{\sqrt{a_1^2+b_1^2+c_1^2} \sqrt{a_2^2+b_2^2+c_2^2}}\)
where θ be the acute angle between given lines
⇒ cos θ = \(\frac{3}{\sqrt{13} \sqrt{14}}=\frac{3}{\sqrt{182}}\)
∴ θ = cos^-1 \(\frac{3}{\sqrt{182}}\).

(iii) The D’ratios of given two lines are < 1, 0, – 1 > and < 3, 4, 5 >
If θ be the required angle between given lines
Then cos θ = \(\frac{|1 \cdot(3)+0(4)-1(5)|}{\sqrt{1+0+1} \sqrt{9+16+25}}\)
cos θ = \(\left|\frac{-2}{\sqrt{2} \times 5 \sqrt{2}}\right|=\left|-\frac{1}{5}\right|=\frac{1}{5}\)
∴ θ = cos^-1 \(\left|\frac{-2}{\sqrt{2} \times 5 \sqrt{2}}\right|=\left|-\frac{1}{5}\right|=\frac{1}{5}\)

Question 5 (old).
Show that the lines x = – y = 2z and x + 2 = 2y – 1 = – z + 1 are perpendicular to each other.
Solution:
Given lines can be written as
\(\frac{x}{1}=\frac{y}{-1}=\frac{z}{\frac{1}{2}}\)
⇒ \(\frac{x}{2}=\frac{y}{-2}=\frac{z}{1}\) ………………..(1)
and \(\frac{x+2}{1}=\frac{y-\frac{1}{2}}{\frac{1}{2}}=\frac{z-1}{-1}\)
\(\frac{x+2}{2}=\frac{y-\frac{1}{2}}{1}=\frac{z-1}{-2}\) ………………………….(2)
Here a₁ = 2 ;
b₁ = – 2
c₁ = 1
a₂ = 1
b₂ = 1
c₂ = – 2
Here, a₁a₂ + b₁b₂ + c₁c₂ = 0
= 2 (2) – 2(1) + 1 (- 2) = 0
∴ lines (1) and (2) are ⊥ to each other.

Question 6.
If the lines \(\frac{x-1}{-3}=\frac{y-2}{2 \lambda}=\frac{z-3}{2}\) and \(\frac{x-1}{3 \lambda}=\frac{y-1}{2}=\frac{z-6}{-5}\) are perpendicualr, find the value of λ. Hence, find whether the lines are intersecting or not.
Solution:
Given equation of lines are
\(\frac{x-1}{-3}=\frac{y-2}{2 \lambda}=\frac{z-3}{2}\) …………………(1)
\(\frac{x-1}{3 \lambda}=\frac{y-1}{2}=\frac{z-6}{-5}\) ………………….(2)
D’s ratio of lines (1) and (2) are ;
< – 3, 2λ, 2 > and < 3λ, 2, – 5 >
Now lines (1) and (2) are perpendicualr to each other.
if (- 3) (3λ) + (2λ) 2 + 2 (- 5) = 0
|a₁a₂ + b₁b₂ + c₁c₂| = 0
if – 9λ + 4λ – 10 = 0
if – 5λ – 10 = 0
if λ = – 2
Thus both given lines (1) and (2) becomes ;
\(\frac{x-1}{-3}=\frac{y-2}{-4}=\frac{z-3}{2}\) = t ……………………..(3)
and \(\frac{x-1}{-6}=\frac{y-1}{2}=\frac{z-6}{-5}\) ……………………(4)
Any point on line (3) be (- 3t + 1, – 4t + 2, 2t + 3) it lies on line (4)
iff \(\frac{-3 t+1-1}{-6}=\frac{-4 t+2-1}{2}=\frac{2 t+3-6}{-5}\) are consistent
iff \(\frac{t}{2}=\frac{-4 t+1}{2}=\frac{2 t-3}{-5}\) are consistent
if t = – 4t + 1 and – 5 (- 4t + 1) = + 2 (2t – 3) are consistent
iff 5t = 1 and 16t = – 1 are consistent
if t = \(\frac{1}{5}\) and t = \(\frac{-1}{16}\) are consistent, which is false
Thus for λ = – 2, both lines do not intersects.

(ii) Find the value of λ so that the lines \(\frac{1-x}{3}=\frac{7 y-14}{\lambda}=\frac{z-3}{2}\) and \(\frac{7-7 x}{3 \lambda}=\frac{y-5}{1}=\frac{6-z}{5}\) areatright angles. Also, find whether the lines are intersecting or not.
Solution:
Given lines can be written as :
\(\frac{7-7 x}{3 \lambda}=\frac{y-5}{1}=\frac{6-z}{5}\) ………………….(1)
\(\frac{x-1}{\frac{3 \lambda}{-7}}=\frac{y-5}{1}=\frac{z-6}{-5}\) ……………..(2)
Thus direction ratios of line (1) and (2) are ;
< – 3, \(\frac{\lambda}{7}\), 2 > and < \(\frac{3 \lambda}{-7}\), 1, – 5 >
Now lines (1) and (2) are ⊥to each other
iff – 3 (\(\frac{3 \lambda}{-7}\)) + \(\frac{\lambda}{7}\) × 1 + 2 (- 5) = 0
iff \(\frac{9 \lambda}{7}+\frac{\lambda}{7}\) – 10 = 0
iff \(\frac{10 \lambda}{7}\) – 10 = 0 iff λ = 7
Then given lines becomes ;
\(\frac{x-1}{-3}=\frac{y-2}{1}=\frac{z-3}{2}\) = t (say) ………………….(3)
and \(\frac{x-1}{-3}=\frac{y-5}{1}=\frac{z-6}{-5}\) ……………………(4)
Any point on line (3) be (- 3t + 1, t + 2, 2t + 3)
it lies on line (4)
iff \(\frac{-3 t+1-1}{-3}=\frac{t+2-5}{1}=\frac{2 t+3-6}{-5}\) are consistent.
iff t = t – 3 = \(\frac{2 t-3}{-5}\) are consistent.
iff t = t – 3 and t – 3 = \(\frac{2 t-3}{-5}\) are consistent
iff 0 = – 3, which is false.
Hence for λ = 7, both given lines are not intersecting.

Question 7.
Find the equations of the perpendicular drawn from the ppint A (2, 4, – 1) to the line \(\frac{x+5}{1}=\frac{y+3}{4}=\frac{z-6}{-9}\).
Solution:
Let P (2, 4, – 1) be the given point
and M be the foot of ⊥ from P on line AB :
\(\frac{x+5}{1}=\frac{y+3}{4}=\frac{z-6}{-9}\) = t (say)

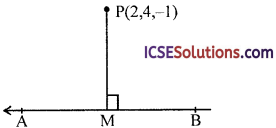
Any point on given line AB is M (t – 5, 4t – 3, – 9t + 6)
∴ D’ratios of line PM are < t – 7, 4t – 7, – 9t + 7 >
Since line PM is perpendicular to line AB.
∴ (t – 7) 1 + (4t – 7) 4 + (- 9t + 7) (- 9) = 0
⇒ t – 7 + 16t – 28 + 81t – 63 = 0
⇒ 98t = 98
⇒ t = 1
∴ Coordinates of foot of perpendicular M is (- 4, 1, – 3).
∴ D’ratios of line PM are < – 4 – 2, 1 – 4, – 3 + 1 >
i.e. <- 6, – 3, – 2 > i.e. < 6, 3, 2 >
∴ eqn. of perpendicular PM in cartesian form is given by \(\frac{x-2}{6}=\frac{y-4}{3}=\frac{z+1}{2}\).

Question 8.
(i) Find the foot of perpendicular drawn from the point (0, 2, 3) on the line \(\frac{x+3}{5}=\frac{y-1}{2}=\frac{z+4}{3}\).
(ii) Find the distance of the point (2, 4, – 1) from the line \(\frac{x+5}{1}=\frac{y+3}{4}=\frac{z-6}{-9}\) (NCERT Exemplar)
Solution:
(i) Given point be P (0, 2, 3) and given line AB is given by
\(\frac{x+3}{5}=\frac{y-1}{2}=\frac{z+4}{3}\) ………………….(1)
Any point on line (1) is say M (5t – 3, 2t + 1, 3t – 4)

Direction ratios of line PM are
< 5t – 3 – 0, 2t + 1 – 2, 3t – 4 – 3 >
i.e. < 5t – 3, 2t – 1, 3t – 7 >
Since line AB is ⊥ to line PM
∴ 5 (5t – 3) + 2 (2t – 1) + 3 (3t – 7) = 0
⇒ 25t – 15 + 4t – 2 + 9t – 21 = 0
⇒ 38t – 38 = 0
⇒ t = 1
∴ Coordinates of foot of ⊥ from P on given line is given by M (2, 3, – 1).

(ii) Let P (2, 4, – 1) be the given point
and M be the foot of ⊥ from P on line AB:
\(\frac{x+5}{1}=\frac{y+3}{4}=\frac{z-6}{-9}\) = t (say)

Any point on given line AB is M (t – 5, 4t – 3, – 9t + 6)
∴ D’ratios of line PM are < t – 7, 4t – 7, – 9t + 7 >
Since line PM is perpendicular to line AB.
∴ (t – 7) 1 + (4t – 7) 4 + (- 9t + 7) (- 9) = 0
⇒ t – 7 + 16t – 28 + 81t – 63 = 0
⇒ 98t = 98
⇒ t = 1
∴ Coordinates of foot of perpendicular M is (- 4, 1, – 3).
Thus required ⊥ distance = |PM|
= \(\sqrt{(-4-2)^2+(1-4)^2+(-3+1)^2}\)
= \(\sqrt{36+9+4}=\sqrt{49}\)
= 7 units.

Question 9.
Find the image of the point P (1, 2, 3) in line \(\vec{r}=6 \hat{i}+7 \hat{j}+7 \hat{k}+\lambda(3 \hat{i}+2 \hat{j}-2 \hat{k})\).
Solution:
Given vector equation of line be,
\(\vec{r}=6 \hat{i}+7 \hat{j}+7 \hat{k}+\lambda(3 \hat{i}+2 \hat{j}-2 \hat{k})\)
∴ eqn. of line through the point (6, 7, 7) and || to the vector whose d’ratios are < 3, 2, – 2 > is given by
\(\frac{x-6}{3}=\frac{y-7}{2}=\frac{z-7}{-2}\) = t (say)
Let N be the foot of ⊥ drawn from point P (1, 6, 3) on given line.
So any point on given line (1) be (3t + 6, 2t + 7, – 2t – 7)

Now we want to find the value oft for which this point becomes N.
∴ D’ numbers of line PN are
< 3t + 6 – 1, 2t + 7 – 2, – 2t + 7 – 3 >
i.e. < 3t + 5, 2t + 5, – 2t + 4 >
Since line PN is ⊥ to given line.
∴ (3t + 5) 3 + (2t + 5) 2 + (- 2t + 4) (- 2) = 0
⇒ 17t + 17 = 0
⇒ t = – 1
The coordinates of foot of ⊥ be N (3, 5, 9).
Let Q (α, β, γ) be the image of point P (1, 2, 3).
Then point N (3, 5, 9) be the mid point of line segment PQ.
\(\frac{\alpha+1}{2}\) = 3 ;
\(\frac{\beta+2}{2}\) = 5 ;
\(\frac{\gamma+3}{2}\) = 9
Let α = 5 ; β = 8 ; γ = 15
Hence, the required image of point P (1, 2, 3) on the given line be (5, 8, 15).

Question 9 (old).
A(0, 6, – 9), B(- 3, – 6, 3) and C (7, 4, – 1) are three points. Find the equations of the line AB. If D is the foot of perpendicular drawn from C to the line AB, find coordinates of the point D.
Solution:
Now D’ratios of given line AB are proportional to < – 3 – 0, – 6 – 6, 3 + 9 >
i.e. < – 3, – 12, 12 > i.e. < 1, – 4, – 4 >
Thus eqn. of line AB which passes through A (0, 6, – 9) and B (- 3, – 6, 3) is given by

Any point on given line be given by
\(\frac{x-0}{1}=\frac{y-6}{4}=\frac{z+9}{-4}\) = t
i.e. x = t,
y = 4t + 6 ;
z = – 4t – 9
∴ The coordinates of point D are (t, 4t + 6, – 4t – 9)
Thus the direction ratios of line CD are proportional to < t – 7, 4t + 2, – 4t – 8 >
also the direction ratios of given line are proportional to, < 1, 4, – 4 >
Since CD ⊥ AB.
∴ (t – 7) + (4t + 2) 4 + (- 4t – 8) (- 4) = 0
⇒ t – 7 + 16t + 8 + 16t + 32 = 0
⇒ 33t = – 33
⇒ t = – 1
Thus the required coordinates of D are (- 1, 2, – 5).

Question 10.
(i) Find the equations of the line passing through the point (2, 1, 3) and perpendicular to the lines \(\frac{x-1}{1}=\frac{y-2}{2}=\frac{z-3}{3}\) and \(\frac{x}{-3}=\frac{y}{2}=\frac{z}{5}\).
(ii) Determine the vector and cartesian equations of the line passing through the point (1, 2, – 4) and perpendicular to the two lines \(\frac{x-8}{3}=\frac{y+19}{-16}=\frac{z-10}{7}\) and \(\frac{x-15}{3}=\frac{y-29}{8}=\frac{z-5}{-5}\).
Solution:
(i) Let the direction ratios of the required line be proportional to a, b, c.
Since the required line is ⊥ to two given lines
a + 2b + 3c = 0 …………………(1)
– 3a + 2b + 5c = 0 ………………..(2)
On solving eqn. (1) and (2) by cross multiplication method, we have
\(\frac{a}{10-6}=\frac{b}{-9-5}=\frac{c}{2+6}\)
⇒ \(\frac{a}{4}=\frac{b}{-14}=\frac{c}{8}\)
i.e. \(\frac{a}{2}=\frac{b}{-7}=\frac{c}{4}\)
∴ direction ratio’s of required line are proportional to 2, – 7, 4.
Hence the eqn. of required line which passes through (2, 1, 3) and having direction ratios proportional to < 2, – 7, 4 > is given by
\(\frac{x-2}{2}=\frac{y-1}{-7}=\frac{z-3}{4}\).

(ii) Let the direction ratios of the required lines be proportional to < a, b, c >.
Since the required line is ⊥ to given two lines whose direction ratios are proportional to
< 3, – 16, 7 > and < 3, 8, 5 >
Thus, 3a – 16b + 7c = 0
and 3a + 8b – 5c = 0
By cross multiplication method, we have
\(\frac{a}{80-56}=\frac{b}{21+15}=\frac{c}{24+48}\)
i.e. \(\frac{a}{24}=\frac{b}{36}=\frac{c}{72}\)
i.e. \(\frac{a}{2}=\frac{b}{3}=\frac{c}{6}\)
Hence the required line passing through the point (1, 2, – 4) and having direction ratio proportional to <2, 3, 6> is given by \(\frac{x-1}{2}=\frac{y-2}{3}=\frac{z+4}{6}\).
Thus the vector eqn. of line passing through point whose position vector \(\vec{a}=\hat{i}+2 \hat{j}-4 \hat{k}\) and parallel to \(\vec{b}=2 \hat{i}+3 \hat{j}+6 \hat{k}\) is given by \(\vec{r}=\vec{a}+\lambda \vec{b}\).
i.e. \(\vec{r}=\hat{i}+2 \hat{j}-4 \hat{k}+\lambda(2 \hat{i}+3 \hat{j}+6 \hat{k})\)

Question 10 (old).
Find the image of the point P (1, 6, 3) in the line \(\frac{x}{1}=\frac{y-1}{2}=\frac{z-2}{3}\). (NCERT Exemplar)
Solution:
Given eqn. of line be,
\(\frac{x}{1}=\frac{y-1}{2}=\frac{z-2}{3}\) = t (say) …………………(1)
Let N be the foot of ⊥ drawn from point P (1, 6, 3) on given line

Any point on line (1) be (t + 0, 2t + 1, 3t + 2)
Now we want to find the value oft for which this any point becomes N.
∴ Direction numbers of line NP are
< t – 1, 2t + 1 – 6, 3t + 2 – 3 >
i.e. < t – 1, 2t – 5, 3t – 1 >
Now line NP is ⊥ to given line (1).
∴ (t – 1) 1 + (2t – 5) 2 + (3t – 1) 3 = 0
⇒ 14t – 14 = 0
⇒ t = 1
∴ The foot of N becomes (1, 3, 5).
Let Q (α, β, γ) be the image of point P (1, 6, 3).
Then N (1, 3, 5) becomes the mid-point of line segment PQ
∴ \(\frac{\alpha+1}{2}\) = 1 ;
\(\frac{\beta+6}{2}\) = 3
and \(\frac{\gamma+3}{2}\) = 5
∴ α = 1,
β = 0,
γ = 7
Hence, the image of point P (1, 6, 3) be the point (1, 0, 7) ¡n the given line.

Question 11.
Find the equation of a line passing through the point (1, 2, – 4) and perpendicular to two lines \(\vec{r}=(8 \hat{i}-19 \hat{j}+10 \hat{k})+\lambda(3 \hat{i}-16 \hat{j}+7 \hat{k})\) and \(\vec{r}=(15 \hat{i}+29 \hat{j}+5 \hat{k})+\mu(3 \hat{i}+8 \hat{j}-5 \hat{k})\).
Solution:
Given equations of lines are;
\(\vec{r}=(8 \hat{i}-19 \hat{j}+10 \hat{k})+\lambda(3 \hat{i}-16 \hat{j}+7 \hat{k})\) ………………………( 1)
and \(\vec{r}=(15 \hat{i}+29 \hat{j}+5 \hat{k})+\mu(3 \hat{i}+8 \hat{j}-5 \hat{k})\) …………………….(2)
Thus, given lines are || to vectors \(\overrightarrow{b_1}=3 \hat{i}-16 \hat{j}+7 \hat{k}\) and \(\overrightarrow{b_2}=3 \hat{i}+8 \hat{j}-5 \hat{k}\)
Let < a, b, c > be the direction ratios of required line.
Since required line is ⊥ to eqns. (1) and (2).
Then 3a – 16b + 7c = 0 …………………(3)
3a + 8b – 5c = 0 ……………(4)
On solving (3) and (4); we have
\(\frac{a}{80-56}=\frac{b}{21+15}=\frac{c}{24+48}\)
i.e. \(\frac{a}{24}=\frac{b}{36}=\frac{c}{72}\)
i.e. \(\frac{a}{2}=\frac{b}{3}=\frac{c}{6}\)
Thus, the direction ratios of required line be < 2, 3, 6 >.
Hence the required line parallel to vector \(\vec{b}=2 \hat{i}+3 \hat{j}+6 \hat{k}\)
Thus, the vector eqn. of line passing through the point position vector \(\vec{a}=\hat{i}+2 \hat{j}-4 \hat{k}\) and || to vector \(\vec{b}=2 \hat{i}+3 \hat{j}+6 \hat{k}\) is given by \(\vec{r}=\vec{a}+\lambda \vec{b}\)
i.e. \(\vec{r}=\hat{i}+2 \hat{j}-4 \hat{k}+\lambda(2 \hat{i}+3 \hat{j}+6 \hat{k})\)
where λ be the parameter.