OP Malhotra Class 12 Maths Solutions Chapter 26 Application of Calculus in Commerce and Economics Ex 26(f)

prehensive S Chand Class 12 Maths Solutions Chapter 26 Application of Calculus in Commerce and Economics Ex 26(f) encourages independent learning.

S Chand Class 12 ICSE Maths Solutions Chapter 26 Application of Calculus in Commerce and Economics Ex 26(f)

Question 1.
(i) The marginal revenue (in thousands of rupees) function for a particular commodity is 5 + 3e^-0.03x, where x denotes the number of units sold. Determine the total revenue from the sale of 100 units.
(ii) Find the total revenue from the sale of 10 units of output (x) from the marginal revenue given by MR = \(\frac { 1 }{ 2 }\)x² – 10x + 100.
(iii) A manufacturers marginal revenue function is \(\frac{d R}{d x}=\frac{2000}{\sqrt{4000+x}}\). If x is in rupecs, find the change in the manufacturer’s total revenue if production is increased from 400 to 900 units.
Solution:
(i) Given MR = 5 + 3e^-0.03x

Thus, the required total revenue is ₹ 595000

(ii) Given MR = \(\frac { 3 }{ 20 }\)x² – 10x + 100 and \(\frac { d }{ dx }\)R(x) = MR
∴ required total revenue from the sale of 10 units of output

(iii) Given MR = \(\frac{d \mathrm{R}}{d x}\) = \(\frac{2000}{\sqrt{4000-x}}\) and MR = \(\frac{d}{d x}\)R(x)
Required change in manufacture’s revenue if production is increased from 400 to 900 units

Question 2.
If the marginal revenue function is given by MR = \(\frac{1}{(x+1)^2}+2\), find the total revenue function. Also, find the total revenue when the price is ₹ 2.20.
Solution:
Given marginal revenue function MR = \(\frac{1}{(x+1)^2}+2\) and MR = \(\frac{d}{d x}\)R(x)

Question 3.
Find the total revenue function and the demand function for the following revenue functions:
(i) MR = 9 – 6x² + 2x
(ii) MR = 9 – 4x²
(iii) MR = 20\(e^{-x / 10}\left(1-\frac{x}{10}\right)\)
(iv) MR = \(\frac{1}{x+1}-5\)
(v) MR = \(\frac{5}{(3 x+4)}-7\)
(vi) MR = log(x + 2)
(vii) MR = a + \(\frac{1}{x+b}\) – \(\frac{x}{(x+b)^2}\)
Solution:
(i) Given MR = 9 – x² + 2x and MR = \(\frac { d }{ dx }\)R(x)

When x = 0; R = 0 ∴ from (1); K = 0
Thus eqn. (1) reduces to; R(x) = 9x – 2x³ + x² which is the required total revenue function
∴ px = 9x – 2x³ + x² ⇒ p = 9 – 2x² + x
which is the required demand function.

(iii)
Since when x = 0; R = 0 ∴ from (1); K = 0
Thus eqn. (1) reduces to; R(x) = 20x e^-x/10
which is the required total revenue function.
Since R(x) = p × x = 20x e^-x/10 ⇒ p = 20e^-x/10 be the required demand function.

when x = 0; R = 0 ∴ K = 0
Thus eqn. (1) redues to; R(x) = log |x + 1| – 5x
which is the required total revenue function
Since R(x) = px ∴ px = log | x + 1| – 5x

which is the required demand function.

(vi) Given MR = log(x + 2) since MR = \(\frac { d }{ dx }\)R(x)

Thus, eqn. (1) reduces to ; R(x) = (x + 2) log (x + 2) – x – 2 log 2
Since p(x) = \(\frac { R(x) }{ x }\) = \(\frac{(x+2) \log (x+2)}{x}\) – 1 – \(\frac{2 \log 2}{x}\)
which is the required demand function.

Given when x = 0; R = 0
∴ from (1); K = 1
Thus eqn. (1) reduces to ; R(x) = ax – \(\frac{b}{x+b}\) + 1
which is the required total revenue function
∴ p(x) = \(\frac{\mathrm{R}(x)}{x}\) = a – \(\frac{b}{x(x+b)}\) + \(\frac{1}{x}\) which is the required demand function.

Question 4.
The marginal revenue function of a monopolist is given as R'(x) = 50 – 0.0002x², where R’ denotes marginal cost and x denotes the quantity produced and sold. It is known that total revenue is zero, when x = 0. Find the market demand function for the commodity.
Solution:

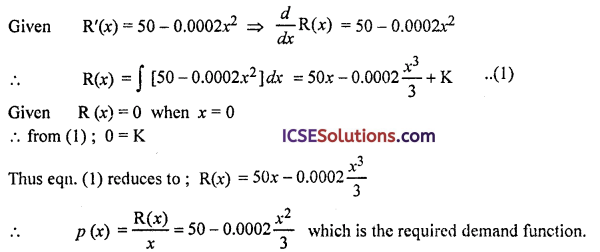
Question 5.
If the marginal revenue function is given by MR = 9 – x² bcing output find total revenue function, average revenue function and demand function.
Solution:
Marginal revenue function (MR) = 9 – x² since MR = \(\frac { d }{ dx }\)R(x)

When x = 0; R(x) = 0 ∴ K = 0
Thus eqn. (1) reduces to ;R(x) = 9x – \(\frac{x^3}{3}\)
∴ p(x) = \(\frac { R(x) }{ x }\) = 9 – \(\frac{x^2}{3}\) which is the required demand function.
Thus average revenue function (AR) = \(\frac { R(x) }{ x }\) = 9 – \(\frac{x^2}{3}\)

Question 6.
The marginal revenue function of a firm is given byMR = \(25 e^{-x / 400}\left(1-\frac{x}{400}\right)\), show that the corresponding demand function is p = 24e\(-x / 400\), where p is the price and x is quantity.
Solution:
Marginal revenue function (MR) = \(25 e^{-x / 400}\left(1-\frac{x}{400}\right)\)
since MR = \(\frac { d }{ dx }\)R(x)

when x = 0 : R(x) = 0 ⇒ K = 0
Then eqn. (1) reduces to; R(x) = 25 × e\(-x / 400\)
Thus p(x) = \(\frac{\mathrm{R}(x)}{x}\) = 25e\(-x / 400\)
which is the required demand function.

Question 7.
A firm has marginal revenue function given by \(M R=\frac{a}{x+b}-c\), where x is the output and a, b, c are constants. Show that the demand law is given by \(M R=\frac{a}{x+b}-c\), where x is the output a, b, c are constants. Show that the demand law is given by \(p=\frac{a}{x} \log \left(\frac{x+b}{b}\right)-c\).
Solution:
Given marginal revenue function (MR) = \(\frac{a}{a+b}-c\) and MR = \(\frac{d}{dx}\)R(x)

Question 8.
If the marginal revenue function of a firm is \(M R=\frac{a b}{(x+b)^2}-c\), find the total revenue function and show that \(p=\frac{a}{x+b}-c\) is the demand function where p is the price, x is the quantity demanded and a, b, c are constants.
Solution:
Marginal revenue function (MR) =

Question 9.
A firm’s marginal revenue function is given by MR = \(\frac{a}{x+b}-\frac{a x}{(x+b)^2}+c\). Show that its demand function is \(p=\frac{a}{x+b}+c\), where a, b, c are constants.
Solution:

since R(x) = 0 when x = 0
∴ from eqn. (1); we have
0 = – a + K ⇒ K = a
∴ from eqn. (1); R(x) = \(-\frac{a b}{x+b}+a+c x\)
∴ R(x) = \(\frac{-a b+a x+a b}{x+b}+c x\) ⇒ R(x) = \([latex]\)[/latex]
∴ \(p(x)=\frac{\mathrm{R}(x)}{x}=\frac{a}{x+b}+c\) which is the required demand function.

Examples

Question 1.
A company is selling a certain product. The demand function of the product is linear. The company can sell 2000 units when the price is ₹ 8 per unit and 3000 units when the price is ₹ 4 per unit. Determine :
(i) the demand function,
(ii) the total revenue function.
Solution:
Let the required demand function be p = a + bx …(1)
given x = 2000 when p = 8
∴ from (1); 8 = a + 2000b …(2)
Also x = 3000 when p = 4
∴ from (1); 4 = a + 3000b …(3)
eqn. (3) – eqn. (2) gives; -4 = 1000 b ⇒ b = \(-\frac{1}{250}\)
∴ from (2); a = 8 – 2000 \(\left(-\frac{1}{250}\right)=16\)
putting the values of a and b in eqn. (1); we have
p = 16 – \(\frac{1}{250}\) x = 16 – 0.004x
Thus total revenue function (R(x)) = px = 16x – 0.004x²

Question 2.
Given the total cost function for x units of a commodity as
\(C(x)=\frac{1}{3} x^3+x^2-8 x+5\) Find:
(i) the marginal cost function,
(ii) the average cost function,
(iii) the slope of average cost function.
Solution:
(i) Given cost function C(x) = \(\frac{x^3}{3}\) + x² – 8x + 5
∴ Marginal cost function (MR) = \(\frac{d}{d x} \mathrm{C}(x)=\frac{d}{d x}\left[\frac{x^3}{3}+x^2-8 x+5\right]=x^2+2 x-8\)
(ii) Average cost function (AC) = \(\frac{C(x)}{x}=\frac{x^2}{3}+x-8+\frac{5}{x}\)
(iii) The slope of average cost function = \(\frac{d}{d x}(\mathrm{AC})=\frac{2 x}{3}+1-\frac{5}{x^2}\)

Question 3.
A firm has the following total cost and demand functions :
\(C(x)=\frac{x^3}{3}-7 x^2+11 x+50 \text { and } p=100-x\)
Find the profit maximizing output.
Solution:
Given cost function C(x) = \(\frac{x^3}{3}\) – 7x² + 111x + 50
and demand function p = 100 – x
∴ Total revenue function = R(x) = px = (100 – x)x
and profit function =P(x) = R(x) – C(x)
∴ P(x) = 100x – x² – \(\frac{x^3}{3}\) + 7x² – 111x – 50
\(\frac{d}{d x} \mathrm{P}(x)\) = – x² + 12x – 11 and \(\frac{d^2}{d x^2} \mathrm{P}(x)\) = -2x + 12
For maxima/minima, \(\frac{d \mathrm{P}}{d x}=0\) ⇒ – x² + 12x – 11 = 0 ⇒ x² – 12x + 11 = 0
⇒ (x – 1)(x – 11) = 0 ⇒ x = 1, 11
and \(\left(\frac{d^2}{d x^2} \mathrm{P}(x)\right)_{x=11}\) = -22 + 12 = – 10 < 0
Thus profit function P(x) is maximum when x = 11
and Maximum profit = P(11) = –\(\frac{11^3}{3}\) + 6 × 11² – 121 – 50
= –\(\frac{1331}{3}\) + 726 – 171 = \(\frac{-1331+2178-513}{3}\) = \(\frac{343}{3}\)

Question 4.
A television manufacture finds that the total cost for the production and marketing of x number of television sets is C(x) = 300x² + 4200x + 13500.
Each product is sold for ₹ 8400. Determine the break-even points.
Solution:
Given cost function C(x) = 300x² + 4200x + 13500
S.P of each product = ₹ 8400
∴ R(x) = Revenue function = 8400 × x where x be the no. of television sets
Thus, Profit function P(x) = R(x) – C(x) = 8400x – 300x² – 4200x – 13500
= 4200x – 300x² – 13500
For break even points; P(x) = 0 ⇒ – 300x² + 4200x – 13500 = 0
⇒ – 300 [x² – 10x + 45] = 0 ⇒ (x – 5)(x – 9) = 0 ⇒ x = 5, 9 units

Question 5.
The fixed cost of a new product is ₹ 18000 and the variable cost is ₹ 550 per unit. If the demand function p(x) = 4000 – 150x, find the break-even points.
Solution:
Given the fixed cost of new product = TFC = ₹ 18000
Variable cost of product = TVC = 550 x where x be the no. of new products
∴ Total cost function C(x) = TFC + TVC ⇒ C(x) = 18000 + 550x
Given demand function p(x) = 4000 – 150x
∴ Revenue function = R(x) = p(x) . x = 4000x – 150x²
thus profit function = P(x) = R(x) – C(x) = 4000x – 150x² – 18000 – 550x
= -150x² + 3450x – 18000
For break even point ; P(x) = 0 ⇒ – 150(x² – 23x + 120) = 0
⇒ (x – 15)(x – 8) =0 ⇒ x = 15, 8 units

Question 6.
The average cost function associated with producing and marketing x units of an item is given by AC = 2x -11 + \(\frac{50}{x}\).
Find :
(i) the total cost function and marginal cost function.
(ii) the range of values of the output x, for which AC is decreasing.
Solution:
Let C(x) be the required total cost function.
Given AC = 2x – 11 + \(\frac{50}{x}\)
since AC = \(\frac{\mathrm{C}(x)}{x}\) ⇒ C(x) = AC × x = 2x² – 11x + 50
∴ Marginal cost function (MC) = \(\frac{d}{d x} \mathrm{C}(x)\) = \(\frac{d}{d x}\left(2 x^2-11 x+50\right)\) = 4x – 11
For AC is to be increasing; \(\frac{d}{d x}(\mathrm{AC})>0 \Rightarrow 4 x-11>0 \Rightarrow x>\frac{11}{4}\)
Thus average cost increasing if the output x > \(\frac{11}{4}\).

Question 7.
The cost of manufacturing of certain items consists of ₹ 1600 as overheads, ₹ 30 per item as the cost of the material and the labour cost ₹\(\frac{x^2}{100}\) for x items produced. How many items must be produced to have a minimum average cost ?
Solution:
Given total fixed cost = TFC = ₹ 1600
Cost of material for x items = ₹ 30x
Total labour cost for x items = ₹ \(\frac{x^2}{100}\)
Thus total cost function = C(x) = TFC + TVC ⇒ C(x) = 1600 + 30x + \(\frac{x^2}{100}\)
∴ average cost function (RC) = \(\frac{C(x)}{x}\) = \(\frac{1600}{x}\) + 30 + \(\frac{x}{100}\)
Now \(\frac{d}{d x}(\mathrm{AC})\) = – \(\frac{1600}{x^2}\) + \(\frac{1}{100}\) and \(\frac{d^2}{d x^2}(\mathrm{AC})\) = \(\frac{3200}{x^3}\)
Now maxima/minima, \(\frac{d}{d x}(\mathrm{AC})\) = 0 ⇒ \(\frac{-1600}{x^2}\) + \(\frac{1}{100}\) = 0
⇒ x² = 16000 = (400)²
⇒ x = 400 (∵ x > 0)
at x = 400; \(\frac{d^2}{d x^2}(\mathrm{AC})=\frac{3200}{(400)^3}>0\)
Thus AC i.e. average cost function is minimise when x = 400 units.
Thus, the required no. of items must be produced to have a minimum average cost be 400 .

Question 8.
The average cost function AC for a commodity is given by AC = x + 5 + \(\frac{36}{x}\) in terms of ouput x. Find the
(i) total cost and the marginal cost as the functions of x.
(ii) output for which AC increases.
Solution:
Given average cost function (AC)
= x + 5 + \(\frac{36}{x}\) since AC = \(\frac{\mathrm{C}(x)}{x}\)
⇒ C(x) = AC × x = x² + 5x + 36
which is the required total cost function.
Now MC = \(\frac{d}{d x}\)C(x) = \(\frac{d}{d x}\) (x² + 5x + 36) = 2x + 5
Now, \(\frac{d}{d x}\)(AC) > 0 ⇒ 1 – \(\frac{36}{x^2}\) > 0
⇒ x² > 36 ⇒ | x | > 6 ⇒ x > 6 [∵ x > 0]
Thus the required output for which AC increases be greater than 6 .

Question 9.
Given that the total cost function for x units of a commodity is
\(C(x)=\frac{x^3}{3}+3 x^2-7 x+16\)
(i) Find the Marginal Cost (MC)
(ii) Find the Average Cost (AC)
(iii) Prove that : Marginal Average Cost
\((M A C)=\frac{x(\mathrm{MC})-\mathrm{C}(x)}{x^2}\)
Solution:
Given C(x) = \(\frac{x^3}{3}\) + 3x² – 7x + 16
(i) Marginal cost function(MC) = \(\frac{d}{d x} \mathrm{C}(x)\) = x² + 6x – 7
(ii) Average cost function (AC) = \(\frac{\mathrm{C}(x)}{x}\) = \(\frac{x^2}{3}\) + 3x – 7 + \(\frac{16}{x}\)
(iii) ∴ Marginal average cost (MAC)

Question 10.
If total cost function is given by C = a + bx + cx², where x is the quantity of output, show that : \(\frac{d}{d x}(A C)=\frac{1}{x}(M C-A C)\), where MC is the marginal cost and AC is the average cost.
Solution:

Question 11.
A company produces a commodity with ₹ 24000 fixed cost. The variable cost is estimated to be 25% of the total revenue recovered on selling the product at a rate of ₹ 8 per unit. Find the following:
(i) cost function
(ii) Revenue function
(iii) Break-even point
Solution:
Given total fixed cost of commodity (TFC) = ₹ 24000
given cost of one unit =₹ 8
∴ Cost of x units = ₹ 8x
Thus, revenue function = R(x) = 8x
∴ Total variable cost(TVC) = \(\frac { 1 }{ 4 }\) × 8x = ₹ 2x
Therefore, total cost function C(x) = TFC + TVC = 24000 + 2x
∴ profit function = P(x) = R(x) – C(x) = 8x -2 4000 – 2x = 6x – 24000
For break even points; P(x) = 0
⇒ 6x – 24000 = 0 ⇒ x = 4000

Question 12.
A firm has the cost function C = \(\frac{x^3}{3}\) – 7x² + 11x + 50 and demand function x = 100 – P.
(i) Write the total revenue function in terms of x.
(ii) Formulate the total profit function P in terms of x.
(iii) Find the profit maximising level of output x.
Solution:
Given cost function
C(x) = \(\frac{x^3}{3}\) – 7x² + 111x + 50
and demand function be given by x =100 – P ⇒ P = 100 – x
(i) ∴ Total revenue function = R(x) = Px =100x – x²
(ii) Total profit function = p(x) = R(x) – C(x)
= 100x – x² – \(\frac{x^3}{3}\) + 7x² – 111x – 50
= \(-\frac{x^3}{3}\) + 6x² – 11x – 50
∴ \(\frac{d}{d x} \mathrm{P}(x)\) = – x² + 12x – 11 and
\(\frac{d^2}{d x^2} \mathrm{P}(x)\) = – 2x + 12
For maxima/minima, we put \(\frac{d}{d x} \mathrm{P}(x)\) = 0
⇒ – (x² – 12x + 11) = 0
⇒ (x – 1) (x – 11) = 0 ⇒ x = 1, 11 at x = 11,
\(\frac{d^2}{d x^2} P(x)\) = – 22 + 12 = – 10 < 0
Thus profit P(x) is maximise when x = 11

Question 13.
The demand function is \(\frac{24-2 p}{3}\) where x is the number of units demanded and p is the price per unit. Find:
(i) The revenue function R in terms of p.
(ii) The price and the number of units demanded for which the revenue is maximum.
Solution:
Given demand function is x = \(\frac { 1 }{ 3 }\) (24 – 2p)
⇒ 3x = 24 – 2p ⇒ p = \(\frac{24-3 x}{2}\) ….(1)
Thus, revenue function R(x) = px = \(\left(12-\frac{3}{2} x\right) x\)
∴ \(\frac{d}{d x} \mathrm{R}(x)\) = 12 – 3x and \(\frac{d^2}{d x^2} \mathrm{R}(x)\) = – 3
For maxima/minima, we put \(\frac{d}{d x} \mathrm{R}(x)\) = 0
⇒ 12 – 3x = 0 ⇒ x = 4

Thus Revenue function R is maximise when x = 4 units
∴ from (1); p = \(\frac{24-12}{2}\) = ₹ 6 and
Revenue function = px = \(\frac{1}{3}\) (24p – 2p²)