ML Aggarwal Class 12 Maths Solutions Section A Chapter 2 Inverse Trigonometric Functions Ex 2.2

Effective ML Aggarwal Class 12 Solutions ISC Chapter 2 Inverse Trigonometric Functions Ex 2.2 can help bridge the gap between theory and application.

ML Aggarwal Class 12 Maths Solutions Section A Chapter 2 Inverse Trigonometric Functions Ex 2.2

Question 1.
Prove the following:
(i) tan^-1 \(\left(\frac{1+x}{1-x}\right)\) = \(\frac{\pi}{4}\) + tan^-1 x, x < 1
(ii) tan^-1 x + cot^-1 (x + 1) = tan^-1 (x² + x + 1)
Solution:
(i) L.H.S = tan^-1 \(\left(\frac{1+x}{1-x}\right)\)
put x = tan θ, Since x < 1
⇒ tan θ < 1
⇒ 0 < θ < \(\frac{\pi}{4}\)
⇒ \(\frac{\pi}{4}<\frac{\pi}{4}+\theta<\frac{\pi}{4}+\frac{\pi}{4}\)
⇒ \(\frac{\pi}{4}<\frac{\pi}{4}+\theta<\frac{\pi}{2}\)
Now L.H.S = tan^-1 \(\left[\frac{1+\tan \theta}{1-\tan \theta}\right]\)
= tan^-1 [tan (\(\frac{\pi}{4}\) + θ)]
= \(\frac{\pi}{4}\) + θ
[∵ tan^-1 (tan x) = x∀ x ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))]
= \(\frac{\pi}{4}\) + tan^-1 x
[∵ tan θ = x
⇒ θ = tan^-1 x]

(ii) We want to prove that
tan^-1 x + cot^-1 (x + 1) = tan^-1 (x² + x + 1)
i.e., tan^-1 (x² + x + 1) – tan^-1 x = cot^-1 (x + 1)
L.H.S. = tan^-1 (x^-1 + x + 1) – tan^-1 x
= tan^-1 \(\left[\frac{x^2+x+1-x}{1+x\left(x^2+x+1\right)}\right]\)
[∵ tan^-1 x – tan^-1 y = tan^-1 \(\left(\frac{x-y}{1+x y}\right)\) if xy > – 1]
i.e., if x (x² + x + 1) > – 1
⇒ x³ + x² + x + 1 > 0
if (x + 1) (x² + 1) > 0
⇒ x + 1 > 0
= tan^-1 \(\left[\frac{x^2+1}{(x+1)\left(x^2+1\right)}\right]\)
= tan^-1 \(\left(\frac{1}{x+1}\right)\)
= cot^-1 (x + 1) [∵ x + 1 > 0]
[∵ cot^-1 x = tan^-1 \(\frac{1}{x}\) if x > 0]
Thus, tan^-1 x + cot^-1 (x + 1) = tan^-1 (x<sup21 + x + 1).

Question 2.
Prove the following (for suitable values of x and y):
(i) tan^-1 √x + tan^-1 √y = tan^-1 \(\frac{\sqrt{x}+\sqrt{y}}{1-\sqrt{x y}}\)
(ii) tan^-1 \(\frac{x+\sqrt{x}}{1-x^{3 / 2}}\) = tan^-1 x + tan^-1 √x
(iii) tan^-1 \(\left(\frac{1}{\sqrt{3}} \tan \frac{x}{2}\right)\) = \(\frac{1}{2} \cos ^{-1}\left(\frac{1+2 \cos x}{2+\cos x}\right)\)
Solution:
(i) Let us take \(\sqrt{x y}\) < 1
⇒ xy < 1 ………….(1)
Let tan^-1 √x = α
⇒ √x = tan α;
α ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))
and tan^-1 √y = β
⇒ √y = tan β;
β ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))
Now, tan (α + β) = \(\frac{\tan \alpha+\tan \beta}{1-\tan \alpha \tan \beta}\)
= \(\frac{\sqrt{x}+\sqrt{y}}{1-\sqrt{x y}}\) ……………(2)
From (1) ; tan α tan β < 1
⇒ \(\frac{\sin \alpha \sin \beta}{\cos \alpha \cos \beta}\) < 1 where α, β ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)) ∴ cos α cos β > 0
⇒ sin α sin β < cos α cos β ⇒ cos (α + β) > 0
⇒ – \(\frac{\pi}{2}\) < α + β < \(\frac{\pi}{2}\)
∴ from (2) ;
(α + β ) = tan^-1 \(\left(\frac{\sqrt{x}+\sqrt{y}}{1-\sqrt{x y}}\right)\)
[∵ tan^-1 (tan x) = x ∀ x ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
⇒ tan^-1 √x + tan^-1 √y = tan^-1 \(\left(\frac{\sqrt{x}+\sqrt{y}}{1-\sqrt{x} \sqrt{y}}\right)\)

(ii) tan^-1 x + tan^-1 √x = tan^-1 \(\left[\frac{x+\sqrt{x}}{1-x \sqrt{x}}\right]\)
= tan^-1 \(\left[\frac{x+\sqrt{x}}{1-x^{3 / 2}}\right]\) if x < 1
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]

(iii) put \(\frac{1}{\sqrt{3}}\) tan \(\frac{x}{2}\) = y
⇒ tan \(\frac{x}{2}\) = √3 y
∴ cos x = \(\frac{1-\tan ^2 \frac{x}{2}}{1+\tan ^2 \frac{x}{2}}\)
= \(\frac{1-(\sqrt{3} y)^2}{1+(\sqrt{3} y)^2}\)
= \(\frac{1-3 y^2}{1+3 y^2}\)
L.H.S. = tan^-1 \(\left(\frac{1}{\sqrt{3}} \tan \frac{x}{2}\right)\)
= tan^-1 y
= \(\frac{1}{2} \cos ^{-1}\left[\frac{1+\frac{2\left(1-3 y^2\right)}{1+3 y^2}}{2+\frac{1-3 y^2}{1+3 y^2}}\right]\)
= \(\frac{1}{2} \cos ^{-1}\left[\frac{3-3 y^2}{3+3 y^2}\right]\)
= \(\frac{1}{2} \cos ^{-1}\left(\frac{1-y^2}{1+y^2}\right)\) ……………..(1)
putting tan^-1 y = θ
⇒ y = tan θ in eqn. (1)
= \(\frac{1}{2} \cos ^{-1}\left(\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta}\right)\)
= \(\frac{1}{2}\) cos^-1 (cos 2θ)
= \(\frac{1}{2}\) × 2θ = θ
= tan^-1 y
Thus, L.H.S. = R.H.S.
∴ tan^-1 \(\left(\frac{1}{\sqrt{3}} \tan \frac{x}{2}\right)\) = \(\frac{1}{2} \cos ^{-1}\left(\frac{1+2 \cos x}{2+\cos x}\right)\)

Question 3.
Write the following functions in simplest form:
(i) tan^-1 \(\left(\sqrt{\frac{1-\cos x}{1+\cos x}}\right)\) (NCERT)
(ii) tan^-1 \(\left(\frac{\cos x-\sin x}{\cos x+\sin x}\right)\) (NCERT)
(iii) tan^-1 \(\left(\frac{2 \sqrt{x}}{1-x}\right)\)
(iv) sin^-1 \(\left(\sqrt{\frac{x}{1+x}}\right)\)
(v) tan^-1 \(\left(\sqrt{\frac{1-x}{1+x}}\right)\)
(vi) cos^-1 (1 – 2x²)
(vii) sec^-1 \(\left(\frac{1}{2 x^2-1}\right)\)
(viii) tan^-1 \(\frac{x}{\sqrt{a^2-x^2}}\) (NCERT)
Solution:
(i) tan^-1 \(\left(\sqrt{\frac{1-\cos x}{1+\cos x}}\right)\)
= tan^-1 \(\left(\sqrt{\frac{2 \sin ^2 \frac{x}{2}}{2 \cos ^2 \frac{x}{2}}}\right)\)
= tan^-1 (tan \(\frac{x}{2}\))
= \(\frac{x}{2}\)
[∵ tan^-1 (tan x) = x ∀ x ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))

(ii) tan^-1 \(\left(\frac{\cos x-\sin x}{\cos x+\sin x}\right)\)
Divide Num and Deno by cos x, We have
= tan^-1 \(\left(\frac{1-\tan x}{1+\tan x}\right)\)
= tan^-1 (tan (\(\frac{\pi}{4}\) – x))
= \(\frac{\pi}{4}\) – x.

(iii) putting √x = tan θ
⇒ θ = tan^-1 √x
∴ tan^-1 \(\left(\frac{2 \sqrt{x}}{1-x}\right)\) = tan^-1 \(\left(\frac{2 \tan \theta}{1-\tan ^2 \theta}\right)\)
= tan^-1 (tan 2θ)
= 2θ
= 2 tan^-1 x

(iv) putting √x = tan θ
⇒ θ = tan^-1 √x
and x = tan² θ
\(\sqrt{\frac{x}{1+x}}=\frac{\tan \theta}{\sqrt{1+\tan ^2 \theta}}\)
= \(\frac{\frac{\sin \theta}{\cos \theta}}{\sec \theta}\)
= sin θ
∴ sin^-1 \(\left(\sqrt{\frac{x}{1+x}}\right)\) = sin^-1 (sin θ)
= θ = tan^-1 √x

(v) putting x = cos θ
⇒ θ = cos^-1 x
∴ tan^-1 \(\left(\sqrt{\frac{1-x}{1+x}}\right)\) = tan^-1 \(\left[\sqrt{\frac{1-\cos \theta}{1+\cos \theta}}\right]\)
= tan^-1 \(\left[\sqrt{\frac{2 \sin ^2 \frac{\theta}{2}}{2 \cos ^2 \frac{\theta}{2}}}\right]\)
= tan^-1 [tan \(\frac{\theta}{2}\)]
= \(\frac{\theta}{2}\)
= \(\frac{1}{2}\) cos^-1 x.

(vi) putting x = sin θ
⇒ θ = sin^-1 x
∴ cos^-1 (1 – 2x²) = cos^-1 (1 – 2 sin² θ)
= cos^-1 (cos 2θ)
= 2θ
= 2 sin^-1 x

(vii) put x = cos θ
⇒ θ = cos^-1 x
∴ sec^-1 \(\left(\frac{1}{2 \cos ^2 \theta-1}\right)\) = cos^-1 (2 cos² θ)
= cos^-1 (cos 2θ)
= 2θ
= 2 cos^-1 x

(viii) put x = a sin θ
⇒ θ = sin^-1 x \(\frac{x}{a}\)
L.H.S. = tan^-1 \(\left(\frac{x}{\sqrt{a^2-x^2}}\right)\)
= tan^-1 \(\left(\frac{a \sin \theta}{\sqrt{a^2-a^2 \sin ^2 \theta}}\right)\)
= tan^-1 \(\left(\frac{a \sin \theta}{a \cos \theta}\right)\)
= tan^-1 (tan θ)
= θ = sin^-1 \(\frac{x}{a}\)

Question 4.
Prove taht : sin (cot^-1 (cos (tan^-1 x))) = \(\sqrt{\frac{x^2+1}{x^2+2}}\)
Solution:
Now cos (tan^-1 x) = cos { cos^-1 (\(\frac{1}{\sqrt{1+x^2}}\))}
= \(\frac{1}{\sqrt{1+x^2}}\)

∴ sin (cot^-1 (cos (tan^-1 x)) = sin (cot^-1 (\(\frac{1}{\sqrt{1+x^2}}\)))

= sin {sin^-1 (\(\frac{\sqrt{1+x^2}}{\sqrt{1+1+x^2}}\))}
= sin {sin^-1 (\(\frac{\sqrt{1+x^2}}{\sqrt{2+x^2}}\))}
= \(\frac{\sqrt{1+x^2}}{\sqrt{2+x^2}}\)
[∵ sin (sin^-1 x) = x ∀ x ∈ [- 1, 1]]

Question 5.
Find the value of tan \(\frac{1}{2}\left(\sin ^{-1} \frac{2 x}{1+x^2}+\cos ^{-1} \frac{1-y^2}{1+y^2}\right)\), |x| < 1, y > 0, xy < 1.
Solution:
[∵ 2 tan^-1 x = sin^-1 \(\frac{2 x}{1+x^2}\) if |x| ≤ 1
and 2 tan^-1 y = cos \(\left(\frac{1-y^2}{1+y^2}\right)\) if y ≥ 0]
Given expression
= tan \(\frac{1}{2}\) (2 tan^-1 x + 2 tan^-1 y)
= tan (tan^-1 x + tan^-1 y)
= tan [tan^-1 {\(\frac{x+y}{1-x y}\)}]
[∵ tan^-1 x + tan^-1 y = tan^-1 (\(\frac{x+y}{1-x y}\)) if xy < 1]
= \(\frac{x+y}{1-x y}\)
[∵ tan (tan^-1 x) = x ∀ x ∈ R]

Question 6.
Prove the following:
(i) tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{2}{11}\) = tan ^-1 \(\frac{3}{4}\) (NCERT)
(ii) tan^-1 \(\frac{1}{7}\) + tan^-1 \(\frac{1}{13}\) = tan^-1 \(\frac{2}{9}\)
(iii) tan^-1 2 – tan^-1 1 = tan^-1 \(\frac{1}{3}\)
(iv) tan^-1 1 + tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{3}\) = \(\frac{\pi}{2}\)
(v) 2 tan^-1 \(\frac{1}{3}\) + tan^-1 \(\frac{1}{7}\) = \(\frac{\pi}{4}\)
(vi) 2 tan^-1 \(\frac{1}{3}\) + cot^-1 4 = tan^-1 \(\frac{16}{13}\)
(vii) tan^-1 \(\frac{3}{4}\) + tan^-1 \(\frac{3}{5}\) – tan^-1 \(\frac{8}{19}\) = \(\frac{\pi}{4}\)
(viii) cot^-1 1 + cot^-1 2 + cot^-1 = \(\frac{\pi}{2}\)
(ix) tan^-1 2 + tan^-1 3 = \(\frac{3 \pi}{4}\)
(x) tan^-1 \(\frac{1}{5}\) + tan^-1 \(\frac{1}{7}\) + tan^-1 \(\frac{1}{3}\) + tan^-1 \(\frac{1}{8}\) = \(\frac{\pi}{4}\)
Solution:
(i) L.H.S. = tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{2}{11}\)
= tan^-1 \(\left(\frac{\frac{1}{2}+\frac{2}{11}}{1-\frac{1}{2} \times \frac{2}{11}}\right)\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan^-1 \(\left(\frac{\frac{1}{2}+\frac{2}{11}}{1-\frac{1}{2} \times \frac{2}{11}}\right)\)
= tan^-1 \(\left(\frac{\frac{15}{22}}{\frac{20}{22}}\right)\)
= tan (\(\frac{3}{4}\))
= R.H.S.

(ii) L.H.S. = tan^-1 \(\frac{1}{7}\) + 2 tan^-1 \(\frac{1}{13}\)
= tan^-1 \(\left[\frac{\frac{1}{7}+\frac{1}{13}}{1-\frac{1}{7} \frac{1}{13}}\right]\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan^-1 \(\left(\frac{\frac{13+7}{91}}{\frac{91-1}{91}}\right)\)
= tan^-1 (\(\frac{20}{90}\))
= tan^-1 (\(\frac{2}{9}\))
= R.H.S

(iii) L.H.S. = tan^-1 2 – tan^-1 1
= tan^-1 \(\left[\frac{2-1}{1+2 \cdot 1}\right]\)
[Here xy = 2 . 1 = 2 > = – 1]
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan (\(\frac{1}{3}\))
= R.H.S.

(iv) L.H.S. = tan^-1 1 + tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{3}\)
= tan^-1 1 + tan^-1 \(\left[\frac{\frac{1}{2}+\frac{1}{3}}{1-\frac{1}{2}-\frac{1}{3}}\right]\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
Here xy = \(=\frac{1}{2} \cdot \frac{1}{3}=\frac{1}{6}\) < 1
= tan^-1 1 + tan^-1 \(\left(\frac{\frac{5}{6}}{\frac{5}{6}}\right)\)
= tan^-1 1 + tan^-1 1
= 2 tan^-1 1
= 2 × \(\frac{\pi}{4}\)
= \(\frac{\pi}{2}\)
= R.H.S.

(v) L.H.S. = tan^-1 \(\frac{1}{7}\) + 2 tan^-1 \(\frac{1}{3}\)

(vi) L.H.S. = 2 tan^-1 \(\frac{1}{3}\) + cot^-1 4
= 2 tan^-1 \(\frac{1}{3}\) + tan^-1 \(\frac{1}{4}\)
[∵ cot^-1 x = tan^-1 \(\frac{1}{x}\) if x > 0]
= tan^-1 \(\left(\frac{2 \cdot \frac{1}{3}}{1-\frac{1}{9}}\right)\) + tan \(\frac{1}{4}\)
[ ∵ 2 tan^-1 x = tan^-1 \(\left(\frac{2 x}{1-x^2}\right)\) if |x| < 1]
= tan^-1 \(\left(\frac{\frac{2}{3}}{\frac{8}{9}}\right)\) + tan^-1 \(\frac{1}{4}\)
= tan^-1 \(\frac{3}{4}\) + tan^-1 \(\frac{1}{4}\)
= tan^-1 \(\left[\frac{\frac{3}{4}+\frac{1}{4}}{1-\frac{3}{4} \times \frac{1}{4}}\right]\)
= tan^-1 \(\left(\frac{1}{1-\frac{3}{16}}\right)\)
= tan^-1(\(\frac{16}{13}\))
[∵ tan^-1 x + tan^-1 y = tan^-1 (\(\frac{x+y}{1-x y}\)) if xy < 1]

(vii) L.H.S. = tan^-1 \(\frac{3}{4}\) + tan^-1 \(\frac{3}{5}\) – tan^-1 \(\frac{8}{19}\)

(viii) cot^-1 1 + cot^-1 2 + cot^-1 3
= tan^-1 1 + tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{3}\)
[∵ cot^-1 x = tan^-1 \(\frac{1}{x}\) if x > 0]
= tan^-1 1 + tan^-1 \(\left(\frac{\frac{1}{2}+\frac{1}{3}}{1-\frac{1}{2} \cdot \frac{1}{3}}\right)\)
[∵ tan^-1 x + tan^-1 x = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1
Here xy = \(\frac{1}{2} \cdot \frac{1}{3}=\frac{1}{6}\) < 1]
= tan^-1 1 + tan^-1 \(\)
= tan^-1 1 + tan^-1 1
= 2 tan^-1 1
= 2 × \(\frac{\pi}{4}\)
= \(\frac{\pi}{2}\)
= R.H.S.

(ix) L.H.S. = tan^-1 2 + tan^-1 3
= π + tan^-1 \(\left(\frac{2+3}{1-2 \cdot 3}\right)\)
[∵ tan^-1 x + tan^-1 y = π + tan^-1 (\(\frac{x+y}{1-x y}\)) if x > 0, y > 0, xy > 1]
[Here xy = 2.3 = 6 > 1]
= π + tan^-1 \(\left(\frac{5}{-5}\right)\)
= π + tan^-1(- 1)
= π – tan^-1 1
[∵ tan^-1 (- x) = – tan^-1 x ∀ x ∈ R]
= π – \(\frac{\pi}{4}\)
= \(\frac{3 \pi}{4}\)
= R.H.S.

(x) L.H.S. = tan^-1 \(\frac{1}{5}\) + tan^-1 \(\frac{1}{7}\) + tan^-1 \(\frac{1}{3}\) + tan^-1 \(\frac{1}{8}\)

Question 7.
Prove the following:
(i) sin^-1 \(\frac{1}{\sqrt{5}}\) + sin^-1 \(\frac{2}{\sqrt{5}}\) = \(\frac{\pi}{2}\)
(ii) cos^-1 \(\frac{4}{5}\) + cos^-1 \(\frac{12}{13}\) = cos^-1 \(\frac{33}{65}\) (NCERT)
(iii) cos^-1 \(\frac{3}{5}\) + sin^-1 \(\frac{12}{13}\) = sin^-1 \(\frac{56}{65}\)
(iv) tan^-1 \(\frac{1}{3}\) + sec^-1 \(\frac{\sqrt{5}}{2}\) = \(\frac{\pi}{4}\)
(v) cos^-1 \(\frac{4}{5}\) + tan^-1 \(\frac{3}{5}\) = tan^-1 \(\frac{27}{11}\)
(vi) cos^-1 \(\frac{5}{\sqrt{41}}\) + cot^-1 \(\frac{4}{5}\) = \(\frac{\pi}{2}\)
(vii) sin^-1 \(\frac{1}{\sqrt{17}}\) + cos^-1 \(\frac{9}{\sqrt{85}}\) = tan^-1 \(\frac{1}{2}\)
(viii) sin^-1 \(\frac{8}{17}\) + cos^-1 \(\frac{4}{5}\) = cot^-1 \(\frac{36}{77}\)
Solution:
(i) L.H.S. = sin^-1 \(\frac{1}{\sqrt{5}}\) + sin^-1 \(\frac{2}{\sqrt{5}}\)

(ii) L.H.S. = cos^-1 \(\frac{4}{5}\) + cos^-1 \(\frac{12}{13}\)
[∵ cos^-1 x + cos^-1 y = cos^-1 (xy – \(\sqrt{1-x^2} \sqrt{1-y^2}\))]
= cos^-1 \(\left[\frac{4}{5} \times \frac{12}{13}-\sqrt{1-\left(\frac{4}{5}\right)^2} \sqrt{1-\left(\frac{12}{13}\right)^2}\right]\)
= cos^-1 \(\left[\frac{48}{65}-\frac{3}{5} \times \frac{5}{13}\right]\)
= cos^-1 \(\left(\frac{33}{65}\right)\)

(iii) L.H.S. = cos^-1 \(\frac{3}{5}\) + sin^-1 \(\frac{12}{13}\)
= sin^-1 \(\frac{4}{5}\) + sin^-1 \(\frac{12}{13}\)

(iv) L.H.S. = tan^-1 \(\frac{1}{3}\) + sec^-1 \(\frac{\sqrt{5}}{2}\)

= tan^-1 \(\frac{1}{3}\) + tan^-1 (\(\frac{1}{2}\))
= tan^-1 \(\left(\frac{\frac{1}{3}+\frac{1}{2}}{1-\frac{1}{3} \cdot \frac{1}{2}}\right)\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan^-1 \(\left(\frac{\frac{5}{6}}{\frac{5}{6}}\right)\)
= tan^-1 1
= \(\frac{\pi}{4}\)
= R.H.S.

(v) L.H.S. = cos^-1 \(\frac{4}{5}\) + tan^-1 \(\frac{3}{5}\)
= tan^-1 (\(\frac{3}{4}\)) + tan^-1 \(\frac{3}{5}\)

= tan^-1 \(\left[\frac{\frac{3}{4}+\frac{3}{5}}{1-\frac{3}{4} \cdot \frac{3}{5}}\right]\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1 Here xy = \(\frac{3}{4} \cdot \frac{3}{5}=\frac{9}{20}\) < 1]
= tan^-1 \(\left[\frac{\frac{15+12}{20}}{\frac{20-9}{20}}\right]\)
= tan^-1 \(\left(\frac{27}{11}\right)\)
= R.H.S.

(vi) L.H.S. = cos^-1 \(\frac{5}{\sqrt{41}}\) + cot^-1 \(\frac{4}{5}\)
= cos^-1 \(\frac{5}{\sqrt{41}}\) + cos^-1 \(\frac{4}{\sqrt{41}}\)

= cos^-1 \(\left[\frac{5}{\sqrt{41}} \cdot \frac{4}{\sqrt{41}}-\sqrt{1-\left(\frac{5}{\sqrt{41}}\right)^2} \sqrt{1-\left(\frac{4}{\sqrt{41}}\right)^2}\right]\)
[∵ cos^-1 x + cos^-1 y = cos^-1 {xy – \(\sqrt{1-x^2} \sqrt{1-y^2}\)}]
= cos^-1 \(\left[\frac{20}{41}-\frac{4}{\sqrt{41}} \times \frac{5}{\sqrt{41}}\right]\)
= cos^-1 \(\left(\frac{20}{41}-\frac{20}{41}\right)\)
= cos^-1 0
= \(\frac{\pi}{2}\)
= R.H.S. [∵ \(\frac{\pi}{2}\) ∈ [0, π]]

(vii) We convert sin^-1 to tan^-1, for this, we construct
p = 1 ; h = \(\sqrt{17}\)
∴ b = \(\sqrt{h^2-p^2}\)
= \(\sqrt{17-1}\)
= 4

We convert cos^-1 to tan^-1, for this, we construct a triangle with
b = 9 ; h = \(\sqrt{85}\)
and p = \(\sqrt{h^2-b^2}\)
= \(\sqrt{85-81}\)
= 2
∴ cos^-1 \(\left(\frac{9}{\sqrt{85}}\right)\) = tan^-1 \(\left(\frac{2}{9}\right)\)

∴ L.H.S. = tan^-1 \(\left(\frac{1}{4}\right)\) + tan^-1 \(\left(\frac{2}{9}\right)\)
= tan^-1 \(\left(\frac{\frac{1}{4}+\frac{2}{9}}{1-\frac{1}{4} \cdot \frac{2}{9}}\right)\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan^-1 \(\left(\frac{\frac{17}{36}}{\frac{34}{36}}\right)\)
= tan^-1 \(\left(\frac{1}{2}\right)\)
= R.H.S.

(viii) To convert sin^-1 to tan^-1
we have p = 8 ; h = 17
∴ b = \(\sqrt{h^2-p^2}\)
= \(\sqrt{289-64}\) = 15

∴ sin^-1 \(\frac{8}{17}\) = tan^-1 (\(\frac{8}{15}\))
and cos^-1 (\(\frac{4}{5}\)) = tan^-1 (\(\frac{3}{4}\))

(vii) (old)
sin^-1 \(\frac{4}{5}\) + cos^-1 \(\frac{2}{\sqrt{5}}\) = cot^-1 \(\frac{2}{11}\)
Solution:
L.H.S. = sin^-1 \(\frac{4}{5}\) + cos^-1 \(\frac{2}{\sqrt{5}}\)
= sin^-1 \(\frac{4}{5}\) + sin^-1 \(\frac{1}{\sqrt{5}}\)

We convert sin^-1 to cot^-1, we make a right angled triangle with p = 11; h = 5√5
∴ b = \(\sqrt{h^2-p^2}\)
= \(\sqrt{125-121}\) = 2

= cot^-1 (\(\frac{2}{11}\))
= R.H.S.

Question 8.
Evaluate :
(i) tan (2 tan^-1 \(\frac{1}{2}\) – cot^-1 3)
(ii) tan (2 tan^-1 \(\frac{1}{5}\) – \(\frac{\pi}{4}\))
Solution:
(i)

(ii) Now 2 tan^-1 \(\frac{1}{5}\) = tan^-1 \(\left(\frac{2 \times \frac{1}{5}}{1-\left(\frac{1}{5}\right)^2}\right)\)
[∵ 2 tan^-1 x = tan^-1 \(\left(\frac{2 x}{1-x^2}\right)\)]
= tan^-1 \(\left(\frac{\frac{2}{5}}{\frac{24}{25}}\right)\)
= tan^-1 \(\left(\frac{5}{12}\right)\)
∴ tan (2 tan^-1 \(\frac{1}{5}\) – \(\frac{\pi}{4}\)) = tan (tan^-1 \(\frac{5}{12}\) – tan^-1 1)
= tan {tan^-1 (\(\frac{\frac{5}{12}-1}{1+\frac{5}{12} \times 1}\))}
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x-y}{1+x y}\right)\) if xy > – 1 Here xy = \(\frac{5}{12}\) > – 1
= tan (tan^-1 (- \(\frac{7}{17}\)))
= – \(\frac{7}{17}\)
[∵ tan (tan^-1 x) = x ∀ x ∈ R]

Question 9.
Find the values of the following:
(i) tan (cos^-1 \(\frac{3}{5}\) + tan^-1 \(\frac{1}{4}\)) (NCERT Exemplar)
(ii) cos (sin^-1 \(\frac{3}{5}\) + sin^-1 \(\frac{5}{13}\))
(iii) cot (cos^-1 \(\frac{4}{5}\) + sin^-1 \(\frac{2}{\sqrt{13}}\))
(iv) sin (2 tan^-1 \(\frac{2}{3}\) + cos (tan^-1 √3) (NCERT Exemplar)
Solution:
(i) First of all, we convert at cos^-1 to tan^-1
For this we construct a triangle with b = 3 and h = 5
∴ p = \(\sqrt{h^2-b^2}\)
= \(\sqrt{25-9}\) = 4
Thus, cos^-1 \(\frac{3}{5}\) = tan^-1 \(\frac{4}{3}\)
∴ tan (cos^-1 \(\frac{3}{5}\) + tan^-1 \(\frac{1}{4}\)) = tan [tan^-1 \(\frac{4}{3}\) + tan^-1 \(\frac{1}{4}\)]
= tan [tan^-1 \(\left\{\frac{\frac{4}{3}+\frac{1}{4}}{1-\frac{4}{3} \times \frac{1}{4}}\right\}\)]

[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan [tan^-1 \(\left(\frac{19}{12} \times \frac{12}{8}\right)\)]
= tan [tan^-1 \(\frac{19}{8}\)]
= \(\frac{19}{8}\)
[∵ tan (tan^-1 x) = x ∀ x ∈ R]

(ii) cos (sin^-1 \(\frac{3}{5}\) + sin^-1 \(\frac{5}{13}\))
= cos [sin^-1 {\(\frac{3}{5} \sqrt{1-\left(\frac{5}{13}\right)^2}+\frac{5}{13} \sqrt{1-\left(\frac{3}{5}\right)^2}\)}]
[∵ sin^-1 x + sin^-1 y = sin^-1 [x \(\sqrt{1-y^2}\) + y \(\sqrt{1-x^2}\)]
= cos [sin^-1 {\(\frac{3}{5} \times \frac{12}{13}+\frac{5}{13} \times \frac{4}{5}\)}]
= cos [sin^-1 \(\frac{56}{65}\)]
Here we construct a right triangle with p = 56 and h = 65
∴ b = \(\sqrt{(65)^2-(56)^2}\)
= \(\sqrt{1089}\) = 33

∴ sin^-1 (\(\frac{56}{65}\)) = cos^-1 (\(\frac{33}{65}\))
∴ given expression = cos (cos^-1 \(\frac{33}{65}\))
= \(\frac{33}{65}\)

(iii) Now cos^-1 \(\frac{4}{5}\) = tan^-1 \(\frac{3}{4}\)

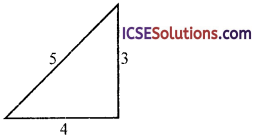
and sin^-1 \(\frac{2}{\sqrt{13}}\) = tan^-1 \(\left(\frac{2}{3}\right)\)

∴ cos^-1 \(\frac{4}{5}\) + sin^-1 \(\frac{2}{\sqrt{13}}\) = tan^-1 \(\frac{3}{4}\) + tan^-1 \(\frac{2}{3}\)
= tan^-1 \(\left[\frac{\frac{3}{4}+\frac{2}{3}}{1-\frac{3}{4} \times \frac{2}{3}}\right]\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan^-1 \(\left(\frac{\frac{17}{12}}{\frac{6}{12}}\right)\)
= tan^-1 \(\frac{17}{6}\)
= cot^-1 \(\frac{6}{17}\)

∴ cot (cos^-1 \(\frac{4}{5}\) + sin^-1 \(\frac{2}{\sqrt{13}}\))
= cot {cot^-1 (\(\frac{6}{17}\))}
= \(\frac{6}{17}\)
[∵ cot (cot^-1 x) = x ∀ x ∈ R]

(iv) To evaluate sin (2 tan^-1 \(\frac{2}{3}\))
= sin [sin^-1 \(\left(\frac{2 \times \frac{2}{3}}{1+\left(\frac{2}{3}\right)^2}\right)\)]
[∵ 2 tan^-1 x = sin^-1 \(\left(\frac{2 x}{1+x^2}\right)\) if | x | ≤ 1]
= sin {sin^-1 \(\left(\frac{\frac{4}{3}}{\frac{13}{9}}\right)\)}
= sin {sin^-1 (\(\frac{12}{13}\))}
= \(\frac{12}{13}\)
[∵ sin (sin^-1 x) = x ∀ x ∈ [- 1, 1]]

To evaluate cos (tan^-1 √3) :
Construct a right triangle with p = √3 ; b = 1
∴ h = \(\sqrt{3+1}\) = 2
∴ tan^-1 √3 = cos^-1 (\(\frac{1}{2}\))

∴ cos (tan^-1 √3) = cos (cos^-1 \(\frac{1}{2}\)) = \(\frac{1}{2}\)
Thus, sin (2 tan^-1 \(\frac{2}{3}\)) – cos (tan^-1 √3)
= \(\frac{12}{13}+\frac{1}{2}\)
= \(\frac{24+13}{26}=\frac{7}{26}\)

Question 10.
(i) tan^-1 \(\left(\frac{2-x}{2+x}\right)\) = \(\frac{1}{2}\) tan^-1 \(\frac{x}{2}\), x > 0
(ii) tan^-1 4x + tan^-1 6x = \(\frac{\pi}{4}\)
(iii) tan^-1 (x + 1) + tan^-1 (x – 1) = tan^-1 \(\frac{6}{17}\)
(iv) tan^-1 \(\left(\frac{x-2}{x-1}\right)\) + tan^-1 \(\left(\frac{x+2}{x+1}\right)\) = \(\frac{\pi}{4}\)
(v) tan^-1 \(\left(\frac{x-2}{x-3}\right)\) + tan^-1 \(\left(\frac{x+2}{x+3}\right)\) = \(\frac{\pi}{4}\)
(vi) tan^-1 \(\left(\frac{x-3}{x-4}\right)\) + tan^-1 \(\left(\frac{x+3}{x+4}\right)\) = \(\frac{\pi}{4}\)
(vii) cos (sin^-1 x) = \(\frac{1}{9}\)
(viii) cos (2 sin^-1 x) = \(\frac{1}{9}\)
(ix) cos^-1 (sin (cos^-1 x)) = \(\frac{\pi}{6}\)
(x) sin^-1 (cos(sin^-1 x)) = \(\frac{\pi}{3}\)
(xi) sin^-1 \(\frac{8}{x}\) + sin^-1 \(\frac{15}{x}\) = \(\frac{\pi}{2}\)
(xii) sin^-1 \(\frac{2 a}{1+a^2}\) + sin^-1 \(\frac{2 b}{1+b^2}\) = 2 tan^-1 x
Solution:
(i) Given that tan^-1 \(\left(\frac{2-x}{2+x}\right)\) = \(\frac{1}{2}\) tan^-1 \(\frac{x}{2}\)
⇒ tan^-1 \(\left(\frac{1-\frac{x}{2}}{1+\frac{x}{2}}\right)\) = \(\frac{1}{2}\) tan^-1 \(\frac{x}{2}\)
⇒ tan^-1 1 – tan \(\frac{x}{2}\) = \(\frac{1}{2}\) tan^-1 \(\frac{x}{2}\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
⇒ \(\frac{\pi}{4}\) = \(\frac{3}{2}\) tan^-1 \(\frac{x}{2}\)
⇒ tan^-1 \(\frac{x}{2}\) = \(\frac{\pi}{6}\)
⇒ \(\frac{x}{2}\) = tan \(\frac{\pi}{6}\)
⇒ \(\frac{x}{2}\) = \(\frac{1}{\sqrt{3}}\)
⇒ x = \(\frac{2}{\sqrt{3}}\)

(ii) Given that tan^-1 4x + tan^-1 6x = \(\frac{\pi}{4}\)
⇒ tan^-1 \(\left(\frac{4 x+6 x}{1-24 x^2}\right)\) = \(\frac{\pi}{4}\) ; (4x) (6x) < 1
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
⇒ tan^-1 \(\left(\frac{10 x}{1-24 x^2}\right)\) = \(\frac{\pi}{4}\)
⇒ \(\frac{10 x}{1-24 x^2}\) = tan \(\frac{\pi}{4}\) = 1;
where x² < \(\frac{1}{24}\)
⇒ 24x² + 10x – 1 = 0; \(-\frac{1}{\sqrt{24}}<x<\frac{1}{\sqrt{24}}\)
⇒ x = \(\frac{-10 \pm \sqrt{100+96}}{48}\) ; \(-\frac{1}{2 \sqrt{6}}<x<\frac{1}{2 \sqrt{6}}\)
⇒ x = \(\frac{-10 \pm 14}{48}\) ; \(-\frac{1}{2 \sqrt{6}}<x<\frac{1}{2 \sqrt{6}}\)
⇒ x = \(-\frac{1}{2}, \frac{1}{12}\) ; \(-\frac{1}{2 \sqrt{6}}<x<\frac{1}{2 \sqrt{6}}\)
But x = \(\frac{1}{12}\) ∈ \(\left(-\frac{1}{2 \sqrt{6}}, \frac{1}{2 \sqrt{6}}\right)\)
Thus the required only solution be x = \(\frac{1}{12}\)

(iii) Given tan^-1 (x + 1) + tan^-1 (x – 1) = tan^-1 \(\frac{6}{17}\)
⇒ tan^-1 \(\left[\frac{x+1+x-1}{1-(x+1)(x-1)}\right]\) = tan^-1 \(\frac{6}{17}\), if (x + 1) (x – 1) < 1
⇒ tan^-1 \(\left[\frac{2 x}{1-x^2+1}\right]\) = tan^-1 \(\frac{6}{17}\) if x² – 1 < 1
⇒ tan^-1 \(\left(\frac{2 x}{2-x^2}\right)\) = tan^-1 \(\frac{6}{17}\) if x² < 2
⇒ \(\frac{2 x}{2-x^2}=\frac{6}{17}\) if |x| < √2
⇒ 6x² + 34x – 12 = 0 if – √2 < x < √2
⇒ 3x² + 17x – 6 = 0 if – √2 < x < √2
∴ x = \(\frac{-17 \pm \sqrt{289+72}}{6}\)
= \(\frac{-17 \pm \sqrt{361}}{6}\)
⇒ x = \(\frac{-17 \pm 19}{6}\) and – √2 < x < √2
⇒ x = – 6, \(\frac{1}{3}\) and – √2 < x < √2
Clearly x = \(\frac{1}{3}\) [∵ x = – 6 ∉ (- √2, √2)]

(iv) Given tan^-1 \(\left(\frac{x-2}{x-1}\right)\) + tan^-1 \(\left(\frac{x+2}{x+1}\right)\) = \(\frac{\pi}{4}\)

⇒ x = ± \(\sqrt{\frac{7}{2}}\); x > 1 or x < – 1 Since both values of x satisfies |x| > 1
Thus, required solutions are x = ± \(\sqrt{\frac{7}{2}}\).

(v) Given tan^-1 \(\left(\frac{x-2}{x-3}\right)\) + tan^-1 \(\left(\frac{x+2}{x+3}\right)\) = \(\frac{\pi}{4}\)
tan^-1 \(\left(\frac{x-2}{x-3}\right)\) + tan^-1 \(\left(\frac{x+2}{x+3}\right)\) = tan^-1 (1)
⇒ tan^-1 \(\left[\frac{\frac{x-2}{x-3}+\frac{x+2}{x+3}}{1-\left(\frac{x-2}{x-3}\right)\left(\frac{x+2}{x+3}\right)}\right]\)
= \(\frac{\pi}{4}\) if \(\left(\frac{x-2}{x-3}\right)\left(\frac{x+2}{x+3}\right)\) < 1
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
⇒ tan^-1 \(\left[\frac{(x-2)(x+3)+(x+2)(x-3)}{x^2-9-\left(x^2-4\right)}\right]\) = \(\frac{\pi}{4}\) and \(\frac{x^2-4}{x^2-9}\) – 1 < 0
⇒ \(\frac{2 x^2-12}{-5}\) = tan \(\frac{\pi}{4}\) = 1 and \(\frac{x^2-4-x^2+9}{x^2-9}\) < 0
⇒ 2x² – 12 = – 5 and \(\frac{5}{x^2-9}\) < 0
⇒ 2x² = 7 and x² – 9 < 0
⇒ x = ± \(\sqrt{\frac{7}{2}}\) and |x| < 3
Here both values of x satisfies |x| < 3.
Thus required solution of given eqn. be ± \(\sqrt{\frac{7}{2}}\).

(vi) tan^-1 \(\left(\frac{x-3}{x-4}\right)\) + tan^-1 \(\left(\frac{x+3}{x+4}\right)\) = \(\frac{\pi}{4}\)
⇒ tan^-1 \(\left[\frac{\frac{x-3}{x-4}+\frac{x+3}{x+4}}{1-\left(\frac{x-3}{x-4}\right)\left(\frac{x+3}{x+4}\right)}\right]\) = \(\frac{\pi}{4}\) ;
\(\left(\frac{x-3}{x-4}\right)\) \(\left(\frac{x+3}{x+4}\right)\) < 1
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
⇒ tan^-1 \(\left[\frac{(x-3)(x+4)+(x+3)(x-4)}{x^2-16-\left(x^2-9\right)}\right]\) = \(\frac{\pi}{4}\) ; \(\frac{x^2-9}{x^2-16}\) – 1 < 0
⇒ tan^-1 \(\left(\frac{2 x^2-24}{-7}\right)\) = \(\frac{\pi}{4}\)
if \(\frac{x^2-9-x^2+16}{x^2-16}\) < 0
⇒ \(\frac{2 x^2-24}{-7}\) = tan \(\frac{\pi}{4}\); \(\frac{7}{x^2-16}\) < 0
⇒ 2x² – 24 = – 7 ; x² – 16 < 0
⇒ 2x² = 17 ; |x| < 4
⇒ x = ± \(\sqrt{\frac{17}{2}}\) ; – 4 < x < 4
Clearly both values of x satisfies – 4 < x < 4.
Thus, reqd. solution are x = ± \(\sqrt{\frac{17}{2}}\).

(vii) Given cos (sin^-1 x) = \(\frac{1}{9}\)
\(\sqrt{1-x^2}\) = \(\frac{1}{9}\)
[∵ cos (sin^-1 x) = \(\sqrt{1-x^2}\)]
On squaring we have
1 – x² = \(\frac{1}{81}\)
⇒ x² = 1 – \(\frac{1}{81}\)
= \(\frac{80}{81}\)
⇒ x = ± \(\sqrt{\frac{80}{81}}\)
= ± \(\frac{4 \sqrt{5}}{9}\)

(viii) Given, cos (2 sin^-1 x) = \(\frac{1}{9}\) ……………(1)
put sin^-1 x = θ
⇒ x = sin θ
∴ from (1) ; cos 2θ = \(\frac{1}{9}\)
⇒ 1 – 2 sin² θ = \(\frac{1}{9}\)
⇒ 1 – 2x² = \(\frac{1}{9}\)
⇒ 2x² = 1 – \(\frac{1}{9}\)
= \(\frac{8}{9}\)
⇒ x² = \(\frac{4}{9}\)
⇒ x = ± \(\frac{2}{3}\)

(ix) Since sin (cos^-1 x) = sin (sin^-1 \(\sqrt{1-x^2}\))

= \(\sqrt{1-x^2}\) if |x| ≤ 1
Given, cos^-1 (sin (cos^-1 x)) = \(\frac{\pi}{6}\)
⇒ \(\sqrt{1-x^2}\) = cos \(\frac{\pi}{6}\) = \(\frac{\sqrt{3}}{2}\)
On squaring ; we have
1 – x² = \(\frac{3}{4}\)
⇒ x² = \(\frac{1}{4}\)
⇒ x = ± \(\frac{1}{2}\)

(x) Given sin^-1 (cos (sin^-1 x)) = \(\frac{\pi}{3}\)
⇒ sin^-1 (cos (cos^-1 \(\sqrt{1-x^2}\))) = \(\frac{\pi}{3}\)
⇒ sin^-1 \(\sqrt{1-x^2}\) = \(\frac{\pi}{3}\)
[∵ cos (cos^-1 x) = x ∀ |x| ≤ 1]
⇒ \(\sqrt{1-x^2}\) = sin \(\frac{\pi}{3}\)
= \(\frac{\sqrt{3}}{2}\)
⇒ 1 – x² = \(\frac{3}{4}\)
⇒ x² = \(\frac{1}{4}\)

⇒ x = ± \(\frac{1}{2}\) ∈ [- 1, 1]

(xi) Given, sin^-1 \(\frac{8}{x}\) + sin^-1 \(\frac{15}{x}\) = \(\frac{\pi}{2}\)
⇒ sin^-1 \(\frac{15}{x}\) = \(\frac{\pi}{2}\) – sin^-1 \(\frac{8}{x}\)
⇒ \(\frac{15}{x}\) = sin \(\left(\frac{\pi}{2}-\sin ^{-1} \frac{8}{x}\right)\)
⇒ \(\frac{15}{x}\) = cos (sin^-1 \(\frac{8}{x}\))
⇒ \(\frac{15}{x}\) = \(\sqrt{1-\left(\frac{8}{x}\right)^2}\)
[∵ cos (sin^-1 x) = \(\sqrt{1-x^2}\)]
⇒ \(\frac{15}{x}=\sqrt{1-\frac{64}{x^2}}\) ; on squaring
⇒ \(\frac{225}{x^2}=1-\frac{64}{x^2}\)
⇒ \(\frac{225}{x^2}+\frac{64}{x^2}\) = 1
⇒ \(\frac{289}{x^2}\) = 1
⇒ x² = 289
⇒ x = ± 17
Since x = – 17 does not satisfies the given equation.
[at x = – 17 ; L.H.S is negative while R.H.S is +ve]
Hence x = 17 be the required solution.

(xii) Given sin^-1 \(\frac{2 a}{1+a^2}\) + sin^-1 \(\frac{2 b}{1+b^2}\) = 2 tan^-1 x ………… (1)
put a = tan θ
⇒ θ = tan^-1 a
and b = tan Φ
⇒ Φ = tan^-1 b
where θ, Φ ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))
∴ from eqn. (1) ; we have
sin^-1 (sin 2θ) + sin^-1 (sin 2Φ) = 2 tan^-1 x
⇒ 2θ + 2Φ = 2 tan^-1 x
⇒ tan^-1 a + tan^-1 b = tan^-1 x
⇒ tan^-1 \(\left(\frac{a+b}{1-a b}\right)\) = tan^-1 x
∴ x = \(\frac{a+b}{1-a b}\)

Question 11.
Evaluate the following:
(i) sin^-1 (sin 2)
(ii) sin^-1 (sin 5)
(iii) tan^-1 (tan 4)
(iv) tan^-1 (tan (- 4))
(v) cos^-1 (cos 10)
Solution:
(i) We know that sin^-1 (sin θ) = θ ∀ θ ∈ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
Now 2 ∉ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
∴ sin^-1 (sin 2) ≠ 2
But π – 2 lies between – \(\frac{\pi}{2}\) and \(\frac{\pi}{2}\)
and sin (π – 2) = sin 2
∴ sin^-1 (sin 2) = sin^-1 {sin (π – 2)} = π – 2.

Aliter :
We know that
sin^-1 (sin θ) = π – θ ∀ θ ∈ [- \(\frac{\pi}{2}\), \(\frac{3 \pi}{2}\)]
Since 2 ∈ [\(\frac{\pi}{2}\), \(\frac{3 \pi}{2}\)]
∴ sin^-1 (sin 2) = π – θ = π – 2

(ii) sin^-1 (sin θ) = θ ∀ θ ∈ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
Here sin^-1 (sin 5) ≠ 5
∵ 5 ∉ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
But 2π – 5 and 5 – 2π lies between – \(\frac{\pi}{2}\) and \(\frac{\pi}{2}\).
∴ sin^-1 (sin 5) = sin^-1 {- sin (2π – 5))
[∵ sin (2π – θ) = – sin θ]
= sin^-1 {sin (5 – 2π)} = 5 – 2π

(iii) We know that tan^-1 (tan θ) = θ
if θ ∈ [- \(\frac{\pi}{2}\), \(\frac{3 \pi}{2}\)]
∴ tan^-1 (tan 4) ≠ 4.
Since 4 ∉ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
Both π – 4 and 4 – π both lies between – \(\frac{\pi}{2}\) and \(\frac{\pi}{2}\).
∴ tan^-1 (tan 4) = tan^-1 {- tan (π – 4)}
[∵ tan (π – θ) = – tan θ]
= – tan^-1 {tan (- (π – 4))}
= tan^-1 {tan (4 – π)} = 4 – π

(iv) We know that tan^-1 (tan θ) = θ ∀ θ ∈ [- \(\frac{\pi}{2}\), \(\frac{3 \pi}{2}\)]
Here tan^-1 (tan (- 4)) ≠ – 4
∵ – 4 ∉ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
But π – 4 and 4 – π lies between – \(\frac{\pi}{2}\) and \(\frac{\pi}{2}\).
∴ tan^-1 (tan (- 4)) = tan^-1 (- tan 4)
= tan^-1 (tan (π – 4))
= π – 4 [∵ tan (π – θ) = – tan θ]

(v) We know that cos^-1 (cos θ) = θ ∀ θ ∈ [0, π]
Here cos^-1 (cos 10) ≠ 10
∵ 10 ∉ [0, π]
But 4π – 10 lies between 0 and π.
i.e., 4π – 10 ∈ [0, π]
∴ cos^-1 (cos 10) = cos^-1 (cos (4π – 10)) = 4π – 10.

Question 11 (old).
Solve for x : cos^-1 (sin (cos^-1 x)) = \(\frac{\pi}{6}\) (ISC 2015)
Solution:
Since sin (cos^-1 x) = sin (sin^-1 \(\sqrt{1-x^2}\))

= \(\sqrt{1-x^2}\) if |x| ≤ 1
Given, cos^-1 (sin (cos^-1 x)) = \(\frac{\pi}{6}\)
⇒ cos^-1 \(\sqrt{1-x^2}\) = \(\frac{\pi}{6}\)
⇒ \(\sqrt{1-x^2}\) = cos \(\frac{\pi}{6}\) = \(\frac{\sqrt{3}}{2}\)
On squaring ; we have
1 – x² = \(\frac{3}{4}\)
⇒ x² = \(\frac{1}{4}\)
⇒ x = ± \(\frac{1}{2}\)

Question 12.
Find the values of:
(i) sin (sin^-1 x + cos^-1 x), |x| ≤ 1
(ii) cot (tan^-1 x + cot^-1 x), x ∈ R (NCERT)
Solution:
(i) sin (sin^-1 x + cos^-1 x) = sin \(\frac{\pi}{2}\) = 1
[∵ sin^-1 x + cos^-1 x = \(\frac{\pi}{2}\) ∀ x ∈ [- 1, 1]]

(ii) cot (tan^-1 x + cot^-1 x) = cot \(\frac{\pi}{2}\) = 0
[∵ tan^-1 x + cot^-1 x = \(\frac{\pi}{2}\) ∀ x ∈ R]

Question 12 (old).
Solve the following equations for x:
(i) tan^-1 (x + 1) + tan^-1 (x – 1) = tan^-1 \(\frac{6}{17}\)
(ii) tan^-1 \(\left(\frac{x-2}{x-3}\right)\) + tan^-1 \(\left(\frac{x+2}{x+3}\right)\) = \(\frac{\pi}{4}\)
(iii) tan^-1 \(\left(\frac{x-2}{x-4}\right)\) + tan^-1 \([\left(\frac{x+2}{x+4}\right)/latex] = [latex]\frac{\pi}{4}\)
(iv) cos (sin^-1 x) = \(\frac{1}{9}\)
(v) cos (2 sin^-1 x) = \(\frac{1}{9}\)
(vi) sin^-1 \(\frac{8}{x}\) + sin^-1 \(\frac{15}{x}\) = \(\frac{\pi}{2}\)
(vii) sin^-1 x + sin^-1 (1 – x) = cos^-1 x, x ≠ 0
(viii) sin^-1 \(\frac{2 a}{1+a^2}\) + sin^-1 \(\frac{2 b}{1+b^2}\) = 2 tan^-1 x.
Solution:
(i) Given tan^-1 (x + 1) + tan^-1 (x – 1) = tan^-1 \(\frac{6}{17}\)
⇒ tan^-1 \(\left[\frac{x+1+x-1}{1-(x+1)(x-1)}\right]\) = tan^-1 \(\frac{6}{17}\) if (x + 1) (x – 1) < 1
⇒ tan^-1 \(\left[\frac{2 x}{1-x^2+1}\right]\) = tan^-1 \(\frac{6}{17}\) if x² – 1 < 1
⇒ tan^-1 \(\left[\frac{2 x}{1-x^2+1}\right]\) = tan^-1 \(\frac{6}{17}\) if x² < 2
⇒ \(\frac{2 x}{1-x^2+1}\) = \(\frac{6}{17}\) if |x| < √2
⇒ 6x² + 34x – 12 = 0 if – √2 < x < √2
⇒ 3x² + 17x – 6 = 0 and – √2 < x < √2
∴ x = \(\frac{-17 \pm \sqrt{289+72}}{6}\)
= \(\frac{-17 \pm \sqrt{361}}{6}\)
⇒ x = \(\frac{-17 \pm \sqrt{19}}{6}\) and – √2 < x < √2
⇒ x = – 6, \(\frac{1}{3}\) and – √2 < x < √2
Clearly x = \(\frac{1}{3}\) [∵ x = – 6 ∉ (- √2, √2)

(ii) Given tan^-1 \(\left(\frac{x-2}{x-3}\right)\) + tan^-1 \(\left(\frac{x+2}{x+3}\right)\) = \(\frac{\pi}{4}\)
⇒ tan^-1 \(\left(\frac{x-2}{x-3}\right)\) + tan^-1 \(\left(\frac{x+2}{x+3}\right)\) = tan^-1 1
⇒ tan^-1 \(\left[\frac{\frac{x-2}{x-3}+\frac{x+2}{x+3}}{1-\left(\frac{x-2}{x-3}\right)\left(\frac{x+2}{x+3}\right)}\right]\) = \(\frac{\pi}{4}\) if \(\left(\frac{x-2}{x-3}\right)\left(\frac{x+2}{x+3}\right)\) < 1
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
⇒ tan^-1 \(\left[\frac{(x-2)(x+3)+(x+2)(x-3)}{x^2-9-\left(x^2-4\right)}\right]\) = \(\frac{\pi}{4}\) and \(\frac{x^2-4}{x^2-9}\) – 1< 0
⇒ \(\frac{2 x^2-12}{-5}\) = tan \(\frac{\pi}{4}\) = 1 and \(\frac{x^2-4-x^2+9}{x^2-9}\) < 0
⇒ 2x² – 12 = – 5 and \(\frac{5}{x^2-9}\) < 0
Here both values of x satisfies |x| < 3.
Thus required solution of given eqn. be ± \(\sqrt{\frac{7}{2}}\).

(iii) Given tan^-1 \(\left(\frac{x-2}{x-4}\right)\) + tan^-1 \([\left(\frac{x+2}{x+4}\right)\) = \(\frac{\pi}{4}\)
⇒ tan^-1 \(\left(\frac{x-2}{x-4}\right)\) = tan^-1 1 – tan^-1 \([\left(\frac{x+2}{x+4}\right)\)
⇒ tan^-1 \(\left(\frac{x-2}{x-4}\right)\) = tan^-1 \(\left(\frac{1-\frac{x+2}{x+4}}{1+\frac{x+2}{x+4}}\right)\)
⇒ tan^-1 \(\left(\frac{x-2}{x-4}\right)\) = tan^-1 \(\left(\frac{x+4-x-2}{x+4+x+2}\right)\)
⇒ tan^-1 \(\left(\frac{x-2}{x-4}\right)\) = tan^-1 \(\left(\frac{2}{2 x+6}\right)\)
⇒ tan^-1 \(\left(\frac{x-2}{x-4}\right)\) = tan^-1 \(\left(\frac{1}{x+3}\right)\)
⇒ \(\frac{x-2}{x-4}=\frac{1}{x+3}\)
⇒ (x – 2) (x + 3) = x – 4
⇒ x² + 3x – 2x – 6 = x – 4
⇒ x² = 2
⇒ x = ± √2

(iv) Given, cos (sin^-1 x) = \(\frac{1}{9}\)
\(\) = \(\frac{1}{9}\)
\(\sqrt{1-x^2}\) = \(\frac{1}{9}\)
[∵ cos (sin^-1 x) = \(\sqrt{1-x^2}\)]
On squaring ; we have
1 – x² = \(\frac{1}{81}\)
⇒ x² = 1 – \(\frac{1}{81}\) = \(\frac{80}{81}\)
⇒ x = ± \(\sqrt{\frac{80}{81}}= \pm \frac{4 \sqrt{5}}{9}\)

(v) Given, cos (2 sin^-1 x) = \(\frac{1}{9}\) ………….(1)
put sin^-1 x = θ
⇒ x = sin θ
⇒ 1 – 2 sin² θ = \(\frac{1}{9}\)
⇒ 1 – 2x² = \(\frac{1}{9}\)
⇒ 2x² = 1 – \(\frac{1}{9}\) = latex]\frac{8}{9}[/latex]
⇒ x² = \(\frac{4}{9}\)
⇒ x = ± \(\frac{2}{3}\)

(vi) Given, sin^-1 \(\frac{8}{x}\) + sin^-1 \(\frac{15}{x}\) = \(\frac{\pi}{2}\)
⇒ sin^-1 \(\frac{15}{x}\) = \(\frac{\pi}{2}\) – sin^-1 \(\frac{8}{x}\)
⇒ \(\frac{15}{x}\) = sin (\(\frac{\pi}{2}\) – sin^-1 \(\frac{8}{x}\))
⇒ \(\frac{15}{x}\) = cos (sin^-1 \(\frac{8}{x}\)))
⇒ \(\frac{15}{x}\) = \(\sqrt{1-\left(\frac{8}{x}\right)^2}\)
[∵ cos (sin^-1 x) = \(\sqrt{1-x^2}\)]
⇒ \(\frac{15}{x}\) = \(\sqrt{1-\frac{64}{x^2}}\) ; on squaring
⇒ \(\frac{225}{x^2}=1-\frac{64}{x^2}\)
⇒ \(\frac{225}{x^2}+\frac{64}{x^2}\) = 1
⇒ \(\frac{289}{x^2}\) = 1
⇒ x² = 289
x = ± 17
Since x = – 17 does not satisfies the given equation.
[at x = – 17 ; L.H.S. is negative while R.H.S. is +ve.]
Hence x = 17 be the required solution.

(vii) Given eqn. be
sin^-1 x + sin^-1 (1 – x) = cos^-1 x
⇒ sin^-1 x + sin^-1 (1 – x) = \(\frac{\pi}{2}\) – sin^-1 x
[∵ sin^-1 x + cos^-1 x = \(\frac{\pi}{2}\) ∀ x ∈ [- 1, 1]]
⇒ sin^-1 (1 – x) = \(\frac{\pi}{2}\) – 2 sin^-1 x
⇒ (1 – x) = sin (\(\frac{\pi}{2}\) – 2 sin^-1 x)
⇒ (1 – x) = cos (2 sin^-1 x)
= 1 – 2x²
[Let sin^-1 x = θ
⇒ x = sin θ
∴ cos 2θ = 1 – 2 sin² θ
= 1 – 2x²]
⇒ 2x² – x = 0
⇒ x (2x – 1) = 0
⇒ x = 0, \(\frac{1}{2}\)
But x ≠ 0
∴ x = \(\frac{1}{2}\)
Since x = \(\frac{1}{2}\) satisfies the given equation.
∴ x = \(\frac{1}{2}\) be the required solution.

(viii) Given sin^-1 \(\frac{2 a}{1+a^2}\) + sin^-1 \(\frac{2 b}{1+b^2}\) = 2 tan^-1 x ……….. (1)
put a = tan θ
⇒ θ = tan^-1 a
and b = tan Φ.
⇒ Φ = tan^-1 b
where θ, Φ ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))
∴ from eqn. (1) ; we have
sin^-1 (sin 2θ) + sin^-1 (sin 2Φ) = 2 tan^-1 x
⇒ 2θ + 2Φ = 2 tan^-1 x
⇒ tan^-1 a + tan^-1 b = tan^-1 x
⇒ tan^-1 \(\left(\frac{a+b}{1-a b}\right)\) = tan^-1 x
∴ x = \(\frac{a+b}{1-a b}\)

Question 13.
Find the values of
(i) cosec (sin^-1 x + cos^-1 x), |x| ≤ 1
(ii) cos (sec^-1 x + cosec^-1 x), |x| ≥ 1
Solution:
(i) Since sin^-1 x + cos^-1 x = \(\frac{\pi}{2}\), |x| ≤ 1
∴ cosec (sin^-1 x + cos^-1 x) = cosec \(\frac{\pi}{2}\) = 1

(ii) cos (sec^-1 x + cosec^-1 x), |x| ≥ 1
= cos (\(\frac{\pi}{2}\))
[∵ sec^-1 x + cosec^-1 x = \(\frac{\pi}{2}\) ∀ x ≥ 1]

Question 14.
Find the values of:
(i) tan^-1 (sin (- \(\frac{\pi}{2}\)))
(ii) cos (tan^-1 \(\frac{3}{4}\))
(iii) tan (cos^-1 \(\frac{8}{17}\)) (NCERT Exampler)
Solution:
(i) tan^-1 (sin (- \(\frac{\pi}{2}\))) = tan^-1 (- 1)
[∵ sin (- \(\frac{\pi}{2}\)) = – sin \(\frac{\pi}{2}\) = – 1]
= tan^-1 (tan (- \(\frac{\pi}{4}\))) = – \(\frac{\pi}{4}\) ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))

(ii) First of all we convert tan^-1 to cos^-1,
we make right angled ∆ with p = 3 ; b = 4
∴ h = \(\sqrt{4^2+3^2}\) = 5

∴ tan^-1 \(\frac{3}{4}\) = cos^-1 \(\frac{4}{5}\)
∴ cos (tan^-1 \(\frac{3}{4}\)) = cos (cos^-1 \(\frac{4}{5}\)) = \(\frac{4}{5}\)
[∵ cos (cos^-1 x) = x, |x| ≤ 1]

(iii) First of all we convert cos^-1 to tan^-1.
Let us construct a right triangle with base b = 8 and hypotenuse h = 17.
∴ perpendicular of right triangle p = 15.

Thus cos^-1 \(\frac{8}{17}\) = tan^-1 \(\frac{15}{8}\)
∴ tan (cos^-1 \(\frac{8}{17}\)) = tan (tan^-1 \(\frac{15}{8}\))
= \(\frac{15}{8}\)
[∵ tan (tan^-1 x) = x, ∀ x ∈ R]

Question 15.
Simplify the following:
(i) tan^-1 \(\left(\frac{\sin x}{1+\cos x}\right)\)
(ii) tan^-1 \(\left(\frac{x}{\sqrt{1-x^2}}\right)\), |x| < 1.
(iii) sin^-1 \(\left(\frac{x}{\sqrt{1+x^2}}\right)\)
(iv) cosec^-1 \(\left(\frac{\sqrt{1+x^2}}{x}\right)\)
(v) cot^-1 \(\left(\frac{x}{\sqrt{1-x^2}}\right)\), |x| < 1
(vi) cosec^-1 \(\left(\frac{1}{\sqrt{1-x^2}}\right)\), |x| < 1
Solution:
(i) tan^-1 \(\left(\frac{\sin x}{1+\cos x}\right)\)
= tan^-1 \(\left(\frac{2 \sin \frac{x}{2} \cos \frac{x}{2}}{2 \cos ^2 \frac{x}{2}}\right)\)
= tan^-1 (tan \(\frac{x}{2}\))
= \(\frac{x}{2}\)

(ii) Let ∆ = tan^-1 \(\left(\frac{x}{\sqrt{1-x^2}}\right)\)
put x = sin θ
⇒ θ = sin^-1 x
Since |x| < 1
⇒ – 1 < x < 1
⇒ – 1< sin θ < 1
⇒ – \(\frac{\pi}{2}\) < θ < \(\frac{\pi}{2}\)
∴ ∆ = tan^-1 \(\left(\frac{\sin \theta}{\sqrt{1-\sin ^2 \theta}}\right)\)
= tan^-1 \(\left(\frac{\sin \theta}{|\cos \theta|}\right)\)
= tan^-1 \(\left(\frac{\sin \theta}{\cos \theta}\right)\)
[∵ θ ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))
∴ cos θ > 0
∴ |cos θ| = cos θ]
= tan^-1 (tan θ) = θ
[∵ θ ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))
= sin^-1 x.

(iii) put x = tan θ
⇒ θ = tan^-1 x
∴ sin^-1 \(\left(\frac{x}{\sqrt{1+x^2}}\right)\) = sin^-1 \(\left(\frac{\tan \theta}{\sqrt{1+\tan ^2 \theta}}\right)\)
= sin^-1 \(\left(\frac{\tan \theta}{\sec \theta}\right)\)
= sin^-1 (sin θ)
= θ
= tan^-1 x

(iv) put x = tan θ
⇒ θ = tan^-1 x
cosec^-1 \(\left(\frac{\sqrt{1+x^2}}{x}\right)\) = cosec^-1 \(\left(\frac{\sqrt{1+\tan ^2 \theta}}{\tan \theta}\right)\)
= cosec^-1 \(\left(\frac{\sec \theta}{\tan \theta}\right)\)
= cosec^-1 (cosec θ)
= θ
= tan^-1 x

(v) put x = cos θ
⇒ θ = cos^-1 x
Since |x| < 1
⇒ – 1 < cos θ < 1
⇒ θ ∈ (0, π)
∴ cot^-1 \(\left(\frac{x}{\sqrt{1-x^2}}\right)\) = cot^-1 \(\left(\frac{\cos \theta}{\sqrt{1-\cos ^2 \theta}}\right)\)
= cot^-1 \(\left(\frac{\cos \theta}{|\sin \theta|}\right)\)
= cot^-1 \(\left(\frac{\cos \theta}{\sin \theta}\right)\)
[∵ θ ∈ (0, π))
⇒ sin θ > 0]
= cot^-1 (cot θ) = θ
= cos^-1 x.

(vi) put x = cos θ
⇒ θ = cos^-1 x
Since |x| < 1
⇒ – 1 < x < 1
⇒ – 1 < cos θ < 1
⇒ θ ∈ (0, π)
∴ cosec^-1 \(\left(\frac{1}{\sqrt{1-x^2}}\right)\) = cosec^-1 \(\left(\frac{1}{\sqrt{1-\cos ^2 \theta}}\right)\)
= cosec^-1 \(\left(\frac{1}{|\sin \theta|}\right)\)
= cosec^-1 \(\left(\frac{1}{\sin \theta}\right)\)
[∵ θ ∈ (0, π))
⇒ sin θ > 0]
= cosec^-1 (cosec θ) = θ
= cos^-1 x.

Question 16.
Solve the following equations for x :
(i) tan^-1 x = sin^-1 \(\left(\frac{1}{\sqrt{2}}\right)\)
(ii) cos (sin^-1 x) = \(\frac{1}{2}\)
(iii) 4 sin^-1 x + cos^-1 x = π
(iv) sin^-1 x – cos^-1 x = \(\frac{\pi}{6}\)
(v) 3 tan^-1 x + cot^-1 x = π. (NCERT Exampler)
(vi) sin^-1 x = cos^-1 \(\left(\frac{\sqrt{3}}{2}\right)\)
(vii) cos^-1 x = sin^-1 (- \(\frac{1}{2}\))
(viii) tan^-1 x^-1 = cot^-1 \(\frac{4}{x}\), x > 0
Solution:
(i) Given tan^-1 x = sin^-1 \(\frac{1}{\sqrt{2}}\)
= tan^-1 (\(\frac{1}{1}\))
⇒ tan^-1 x = \(\frac{\pi}{4}\)
⇒ x = tan \(\frac{\pi}{4}\) = 1

(ii) cos (sin^-1 x) = \(\frac{1}{2}\)

⇒ \(\sqrt{1-x^2}\) = \(\frac{1}{2}\) ;
On Squaring ; we have
1 – x² = \(\frac{1}{4}\)
⇒ x² = 1 – \(\frac{1}{4}\)
= \(\frac{3}{4}\)
⇒ x = ± \(\frac{\sqrt{3}}{2}\)

(iii) Given 4 sin^-1 x = π – cos^-1
⇒ 4 (\(\frac{\pi}{2}\) – cos^-1 x) = π – cos^-1
[∵ sin^-1 x + cos^-1 x = \(\frac{\pi}{2}\) ∀ x ∈ [- 1, 1]]
⇒ 2π – 4 cos^-1 x = π – cos^-1 x
⇒ 3 cos^-1 x = π
⇒ cos^-1 x = \(\frac{\pi}{3}\)
⇒ x = cos \(\frac{\pi}{3}\) = \(\frac{1}{2}\)

(iv) Given sin^-1 x – cos^-1 x = \(\frac{\pi}{6}\) …………(1)
Also, sin^-1 x + cos^-1 x = \(\frac{\pi}{2}\) …………(2)
On adding (1) and (2) ; we have
2 sin^-1 x = \(\frac{2 \pi}{3}\)
⇒ sin^-1 x = \(\frac{\pi}{3}\)
[∵ \(\frac{\pi}{3}\) ∈ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
∴ x = sin \(\frac{\pi}{3}\) = \(\frac{\sqrt{3}}{2}\)

(v) Given 3 tan^-1 x + cot^-1 x = π
⇒ 3 tan^-1 x + \(\frac{\pi}{2}\) – tan^-1 x = π
[∵ tan^-1 x + cot^-1 x = \(\frac{\pi}{2}\) ∀ x ∈ R]
⇒ 2 tan^-1 x = π – \(\frac{\pi}{2}\)
= \(\frac{\pi}{2}\)
⇒ tan^-1 x = \(\frac{\pi}{4}\)
⇒ x = tan \(\frac{\pi}{4}\) = 1

(vi) Given sin^-1 x = cos^-1 \(\left(\frac{\sqrt{3}}{2}\right)\)
= cos^-1 (cos \(\frac{\pi}{6}\))
= \(\frac{\pi}{6}\)
[∵ cos^-1 (cos x) = x ∈ (0, π)]
⇒ x = sin \(\frac{\pi}{6}\)
= \(\frac{1}{2}\)

(vii) Given cos^-1 x = sin^-1 (- \(\frac{1}{2}\))
= sin^-1 (sin (- \(\frac{\pi}{6}\)))
= – \(\frac{\pi}{6}\)
[∵ sin^-1 (sin x) = x ∀ x ∈ [- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
⇒ cos^-1 x = – \(\frac{\pi}{6}\)
⇒ cos (cos^-1 x) = cos (- \(\frac{\pi}{6}\))
= cos \(\frac{\pi}{6}\)
⇒ x = \(\frac{\sqrt{3}}{2}\)

(viii) Given tan^-1 x^-1 = cot^-1 \(\frac{4}{x}\), x > 0
⇒ tan^-1 \(\frac{1}{x}\) = cot^-1 \(\frac{4}{x}\), x > 0
⇒ cot^-1 x = cot^-1 \(\frac{4}{x}\)
⇒ x = \(\frac{4}{x}[/latex
⇒ x² = 4
⇒ x = ± 2 but x > 0
∴ x = 2.

Question 17.
If sin (sin^-1 [latex]\frac{2}{3}\) + cos^-1 x) = 1, then find the value of x.
Solution:
Given sin (sin^-1 \(\frac{2}{3}\) + cos^-1 x) = 1
⇒ sin^-1 \(\frac{2}{3}\) + cos^-1 x = sin^-1 1
= \(\frac{\pi}{2}\)
⇒ sin^-1 \(\frac{2}{3}\) = \(\frac{\pi}{2}\) – cos^-1 x = sin^-1 x
⇒ x = \(\frac{2}{3}\)

Question 18.
Find the values of :
(i) tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{3}\)
(ii) cot^-1 2 + cot^-1 3
Solution:
(i) tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{3}\) = tan^-1 \(\left(\frac{\frac{1}{2}+\frac{1}{3}}{1-\frac{1}{2} \cdot \frac{1}{3}}\right)\)
= tan^-1 \(\left(\frac{\frac{5}{6}}{\frac{5}{6}}\right)\)
[∵ tan^-1 x + tan^-1 y = tan^-1 \(\left(\frac{x+y}{1-x y}\right)\) if xy < 1]
= tan^-1 1 = \(\frac{\pi}{4}\)

(ii) cot^-1 2 + cot^-1 3 = tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{3}\)
[∵ cot^-1 x = tan^-1 \(\frac{1}{x}\) if x > 0]
= tan^-1 \(\left[\frac{\frac{1}{2}+\frac{1}{3}}{1-\frac{1}{2} \cdot \frac{1}{3}}\right]\)
= tan^-1 \(\left(\frac{\frac{5}{6}}{\frac{5}{6}}\right)\)
= tan^-1 t
= tan^-1 (tan \(\frac{\pi}{4}\))
= \(\frac{\pi}{4}\)
[∵ tan^-1 (tan x) = x ∀ x ∈ (- \(\frac{\pi}{2}\), \(\frac{\pi}{2}\))]

Question 19.
If tan^-1 x – tan^-1 y = \(\frac{\pi}{4}\), xy > – 1, then find the value of x – y – xy.
Solution:
Given tan^-1 x – tan^-1 y = \(\frac{\pi}{4}\)
⇒ tan^-1 \(\left(\frac{x-y}{1+x y}\right)\) = \(\frac{\pi}{4}\)
[∵ xy > – 1]
⇒ \(\frac{x-y}{1+x y}\) = tan \(\frac{\pi}{4}\) = 1
⇒ x – y = 1 + xy
⇒ x – y – xy = 1

Question 20.
(i) If tan^-1 x = \(\frac{\pi}{10}\) for some x ∈ R, find the value of cot^-1 x. (NCERT Exampler)
(ii) If sin^-1 x + sin^-1 y = \(\frac{\pi}{2}\), then find the value of cos^-1 x + cos^-1 y. (NCERT Exampler)
(iii) If sec^-1 x + sec^-1 y = \(\frac{2 \pi}{3}\), then find the value of cosec^-1 x + cosec^-1 y.
Solution:
(i) Given tan^-1 x = \(\frac{\pi}{10}\)
We know that
tan^-1 x + cot^-1 x = \(\frac{\pi}{2}\) ∀ x ∈ R
⇒ \(\frac{\pi}{10}\) + cot^-1 x = \(\frac{\pi}{2}\)
⇒ cot^-1 x = \(\frac{\pi}{2}-\frac{\pi}{10}\)
= \(\frac{5 \pi-\pi}{10}\)
= \(\frac{4 \pi}{10}=\frac{2 \pi}{5}\)

(ii) Given sin^-1 x + sin^-1 y = \(\frac{\pi}{2}\) ……..(1)
[∵ sin^-1 x + cos^-1 x = \(\frac{\pi}{2}\) ∀ x ∈ [- 1, 1]]
Thus from (1) : we have
\(\frac{\pi}{2}\) – cos^-1 x + \(\frac{\pi}{2}\) – cos^-1 y = \(\frac{\pi}{2}\)
⇒ cos^-1 x + cos^-1 y = π – \(\frac{\pi}{2}\) = \(\frac{\pi}{2}\)

(iii) Given sec^-1 x + sec^-1 y = \(\frac{2 \pi}{3}\)
We know that,
sec^-1 x + cosec^-1 x = \(\frac{\pi}{2}\) if |x| ≥ 1
⇒ \(\frac{\pi}{2}\) – cosec^-1 x + \(\frac{\pi}{2}\) – cosec^-1 y = \(\frac{2 \pi}{3}\)
⇒ cosec^-1 x + cosec^-1 y = \(\frac{\pi}{2}\) – \(\frac{2 \pi}{3}\)
= \(\frac{\pi}{3}\)

Question 21.
If cos^-1 x + cos^-1 y + cos^-1 z = 3π, then find the value of x¹⁰⁰ + y¹⁰⁰ + z¹⁰⁰ – \(\frac{9}{x^{101}+y^{101}+z^{101}}\).
Solution:
Since 0 ≤ cos^-1 x ≤ π,
so maximum value of cos^-1 x is π.
∴ cos^-1 x + cos^-1 y + cos^-1 z = 3π
Thus cos^-1 x = π ;
cos^-1 y = π ;
cos^-1 z = π
⇒ x = cos π = – 1 ;
y = cos π = – 1;
z = cos π = – 1
x¹⁰⁰ + y¹⁰⁰ + z¹⁰⁰ – \(\frac{9}{x^{101}+y^{101}+z^{101}}\) = (- 1)¹⁰⁰ + (- 1)¹⁰⁰ + (- 1)¹⁰⁰ – \(\frac{9}{(-1)^{101}+(-1)^{101}+(-1)^{101}}\)
= 1 + 1 + 1 – \(\frac{9}{-1-1-1}\)
= 1 + 1 + 1 + 3 = 6