Interactive ISC Mathematics Class 12 Solutions Chapter 10 Probability Ex 10.6 engage students in active learning and exploration.
ML Aggarwal Class 12 Maths Solutions Section A Chapter 10 Probability Ex 10.6
Question 1.
A random variable X has the following probability distribution :

(i) Determine the value of a.
(ii) Find P (X < 3), P (X ≥ 4), P (0 < X < 5).
Answer:
Since the random variable X has probability distribution
(i) Σpi = 1
⇒ P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3)+ P(X = 4) + P(X = 5) + P(X = 6) + P(X = 7) = 1
⇒ a + 4a + 3a + la + 8a + 10a + 6a + 9a – 1
⇒ 48a = 1
⇒ a = \(\frac{1}{48}\)
(ii) P(X < 3) = P(X = 0) + P(X = 1) + P(X = 2)
= a + 4a + 3a = 8a
= \(\frac{8}{48}=\frac{1}{6}\)
P (X ≥ 4) = P (X = 4) + P (X = 5) + P (X = 6) + P (X = 7)
= 8a + 10a + 6a + 9a = 33a
= \(\frac{33}{48}=\frac{11}{16}\)
and P (0 < X < 5) = P (X = 1) + P (X = 2) + P (X = 3) + P (X = 4)
= 4a + 3a + 7a + 8a = 22 a
= \(\frac{22}{48}=\frac{11}{24}\)
Question 2.
A random variable X has the probability distribution :

Answer:
E = {X is a prime number} = {2, 3, 5, 7}
F = {X < 4} = {1, 2, 3}
∴ E ∪ F = {1, 2, 3, 5, 7}
Thus P(E ∪ F) = P(1) + P(2) + P (3) + P (5) + P (7)
= 0.15 + 0.23 + 0.12 + 0.2 + 0.07
= 0.77
Question 3.
A random variable X has the following probability distribution where k is some number :

(i) Determine the value of k.
(ii) Find P (X <2), P (X ≤ 2), P (X ≥ 2). (NCERT)
Answer:

We know that otherwise Σpi = 1
⇒ k + 2k + 3k + 0 = 1
⇒ 6k = 1
⇒ k = \(\frac{1}{6}\)
(ii) P (X < 2) = P (X = 0) + P (X = 1)
= k + 2k = 3k = \(\frac{3}{6}=\frac{1}{2}\)
P (X ≤ 2) = P (X = 0) + P (X = 1) + P (X = 2)
= k + 2k + 3k = 6k = \(\frac{6}{6}\) = 1 .
P (X ≥ 2) = 1 – P (X < 2) = 1 – \(\frac{1}{2}=\frac{1}{2}\)
![]()
Question 4.
A coin is tossed twice (or two coins are tossed simultaneously).
(i) Find the probability distribution of X, the number of heads. (NCERT)
(ii) If a random variable Y is defined on this sample space as the number of tails, then find the probability distribution of Y.
Answer:
(i) When a coin is tossed twice
Then sample space = {HH, HT, TH, TT} and all 4 events are equally likely.
Let random variable X be defined as number of heads
P (X = 0) = P (TT) = \(\frac{1}{4}\)
P(X= 1) = P(HT,TH) = \(\frac{2}{4}=\frac{1}{2}\)
P (X = 2) = P (HH) = \(\frac{1}{4}\)
Hence the required probability distribution of X is given below :
![]()
(ii) Let Y be the random variable which is the number of tails. So Y takes value 0, 1, 2
S = {HT, TH, TT, HH}
∴ P (Y = 0) = P (HH) = \(\frac{1}{4}\);
P (Y = 1) = P (HT, TH) = \(\frac{2}{4}\)
P (Y = 2) = P (TT) = \(\frac{1}{4}\)
∴ Probability distribution of Y is given by

Question 5.
A die is rolled. If a random variable X is defined as the number on the upper face, then find its probability distribution.
Answer:
When a die is rolled
Then sample space = {1, 2, 3, 4, 5, 6}
Let X be the random variable denotes the number on the upper face.
X takes values from 1 to 6.
P (X = 1) = \(\frac{1}{6}\) = P (X = 2) = P (X = 3)
= P (X = 4) = P (X = 5)
= P (X = 6)
Question 6.
An urn contains 5 white and 3 black balls. If two balls are drawn at random without replacement and X denotes the number of white balls drawn, then find its probability distribution.
Answer:
Number of white balls in a box = 5
No. of black balls in a box = 3
∴ Total no. of balls in a box = 5 + 3 = 8
Here, X be the random variable denote the number of white balls drawn in two draws of ball.
Then X can take values 0, 1, 2.
P (X = 0) = P (drawing no white balls)
= P (drawing 2 black balls)
= \(\frac{{ }^3 C_2}{{ }^8 C_2}=\frac{3 \times 2}{8 \times 7}=\frac{3}{28}\)
P (X = 1) = P (drawing one white ball and 1 black ball)
= \(\frac{{ }^5 \mathrm{C}_1 \times{ }^3 \mathrm{C}_1}{{ }^8 \mathrm{C}_2}=\frac{5 \times 3 \times 2}{8 \times 7}=\frac{15}{28}\)
P(X = 2) = P (drawing 2 white balls)
= \(\frac{{ }^5 \mathrm{C}_2}{{ }^8 \mathrm{C}_2}=\frac{5 \times 4}{8 \times 7}=\frac{5}{14}\)
required probability distribution of X is given by

![]()
Question 7.
Two cards are drawn simultaneously from a well-shuffled pack of 52 cards. Find the probability distribution of the number of jacks.
Answer:
Let X denotes the number of aces in 2 draws from a pack of 52 cards
∴ X can take values 0, 1,2.
P(X = 0) = probability of getting no ace = probability of getting two other cards
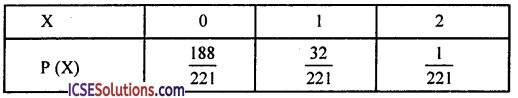
= \(\frac{{ }^{48} C_2}{{ }^{52} C_2}=\frac{48 \times 47}{52 \times 51}=\frac{12 \times 47}{13 \times 51}=\frac{188}{221}\)
P(X = 1) = probability of getting one ace and one other card
= \(\frac{{ }^4 C_1 \times{ }^{48} C_1}{{ }^{52} C_2}=\frac{4 \times 48 \times 2}{52 \times 51}=\frac{32}{221}\)
P(X = 2) = probability of getting two ace card
= \(\frac{{ }^4 C_2}{{ }^{52} C_2}=\frac{4 \times 3}{52 \times 51}=\frac{1}{221}\)
Thus the probability distribution of random variable X is given as under :
Question 8.
Four bad oranges are mixed accidentally with 16 good oranges. Find the probability distribution of the number of bad oranges in a draw of two oranges.
Answer:
Total no. of bad oranges = 4
Total no. of good oranges 16
∴ Total no. of oranges 4 ÷ 16 = 20
Let X denotes the number of bad oranges in a draw of 2 oranges. So X can take values 0, 1, 2.
2 oranges can be drawn simultaneously in 20C2 ways.
∴ P (X = 0) = P (drawing no bad orange) = P (drawing 2 good oranges)
= \(\frac{{ }^{16} C_2}{{ }^{20} C_2}=\frac{16 \times 15}{20 \times 19}=\frac{12}{19}\)
P (X = 1) = P (drawing one bad and one good orange)
= \(\frac{{ }^{16} C_2}{{ }^{20} C_2}=\frac{16 \times 15}{20 \times 19}=\frac{12}{19}\)
P (X = 2) = P (drawing 2 bad oranges)
= \(\frac{{ }^4 \mathrm{C}_2}{{ }^{20} \mathrm{C}_2}=\frac{4 \times 3}{20 \times 19}=\frac{3}{95}\)
The probability distribution of X is given below

Question 9.
Three balls are drawn one by one without replacement from a bag containing 5 white and 4 red balls. Find the probability distribution of the number of white balls drawn.
Answer:
Total no. of white balls = 5
Total no. of red balls = 4
∴ Total no. of balls = 5 + 4 = 9
Let X denote the random variable and denote the number of white balls drawn in a draw of three balls one by one without replacement.
∴ X can takes values 0, 1, 2, 3.
P(X = 0) = P (drawing no white ball)
= P (drawing three red balls)
= \(\frac{{ }^4 C_3}{{ }^9 C_3}=\frac{4 \times 3 \times 2}{9 \times 8 \times 7}=\frac{1}{21}\)
P(X = 1) = P (drawing 1 white ball and 2 black balls)
= \(\frac{{ }^5 \mathrm{C}_1 \times{ }^4 \mathrm{C}_2}{{ }^9 \mathrm{C}_3}=\frac{5 \times 4 \times 3 \times 6}{2 \times 9 \times 8 \times 7}=\frac{5}{14}\)
P(X = 2) = P (drawing 2 white balls and 1 black ball)
= \(\frac{{ }^5 \mathrm{C}_2 \times{ }^4 \mathrm{C}_1}{{ }^9 \mathrm{C}_3}=\frac{5 \times 4 \times 4 \times 6}{2 \times 9 \times 8 \times 7}=\frac{10}{21}\)
P(X = 3) = P (drawing 3 white balls) ‘
= \(\frac{{ }^5 C_3}{{ }^9 C_3}=\frac{5 \times 4 \times 6}{2 \times 9 \times 8 \times 7}=\frac{5}{42}\)
The probability distribution of random variable X is given by

Question 10.
A box contains 12 bulbs of which 3 are defective. A sample of 3 bulbs is selected from the ^^box. Let X denote the number of defective bulbs in the sample. Find the probability distribution of X.
Answer:
Total no. of bulbs = 12
no. of defective bulbs = 3
and no. of good bulbs = 12 – 3 = 9
Let X denote the no. of defective bulbs in a sample of 3 bulbs.
So X can take values 0, 1, 2, 3.
P (X = 0) = P (drawing no defective bulb = P (drawing 3 good bulbs)
= \(\frac{{ }^9 \mathrm{C}_3}{{ }^{12} \mathrm{C}_3}=\frac{9 \times 8 \times 7}{12 \times 11 \times 10}=\frac{42}{110}=\frac{21}{55}\)
P (X = 1) = P (drawing one defective and 2 non-defective bulbs)
= \(\frac{{ }^3 \mathrm{C}_1 \times{ }^9 \mathrm{C}_2}{{ }^{12} \mathrm{C}_3}=\frac{3 \times 9 \times 8 \times 6}{2 \times 12 \times 11 \times 10}=\frac{54}{110}=\frac{27}{55}\)
P (X = 2) = P (drawing two defective and one non-defective bulb)
= \(\frac{{ }^3 \mathrm{C}_2 \times{ }^9 \mathrm{C}_1}{{ }^{12} \mathrm{C}_3}=\frac{3 \times 9}{\frac{12 \times 11 \times 10}{6}}=\frac{3 \times 9 \times 6}{12 \times 11 \times 10}=\frac{27}{220}\)
P (X = 3) = P (drawing three defective bulbs)
= \(\frac{{ }^3 C_3}{{ }^{12} C_3}=\frac{1}{\frac{12 \times 11 \times 10}{6}}=\frac{1}{220}\)
Thus, the required probability distribution of X is given by

![]()
Question 11.
Four bad eggs are mixed with 10 good ones. If 3 eggs are drawn one by one without replacement, then find the probability distribution of the number of bad eggs drawn.
Answer:
Let X denotes the random variable denotes the no. of bad eggs.
∴ X takes values 0, 1, 2, 3.
No. of good eggs = 10
and No. of bad eggs = 4
P(X = 0) =P(No bad egg) = \(\frac{10}{14} \times \frac{9}{13} \times \frac{8}{12}=\frac{30}{91}\)
P (X = 1) = P (one bad egg = P (SFF, FSF, FFS)
= \(\frac{4}{14} \times \frac{10}{13} \times \frac{9}{12}+\frac{10}{14} \times \frac{4}{10} \times \frac{9}{12}+\frac{10}{14} \times \frac{9}{13} \times \frac{4}{12}\)
= \(\frac{3 \times 10 \times 9 \times 4}{14 \times 13 \times 12}=\frac{45}{91}\)
P (X = 2) = P (two badd eggs) = P (SSF, SFS, FSS)
= \(\frac{4}{14} \times \frac{3}{13} \times \frac{10}{12}+\frac{4}{14} \times \frac{10}{13} \times \frac{3}{12}+\frac{10}{14} \times \frac{4}{13} \times \frac{3}{12}\)
= 3 × \(\frac{4 \times 3 \times 10}{14 \times 13 \times 12}=\frac{15}{91}\)
P (X = 3) = P (three bad eggs) = \(\frac{4}{14} \times \frac{3}{13} \times \frac{2}{12}=\frac{1}{91}\)
Required probability distribution of X is given by

Question 12.
Four cards are drawn simultaneously from a well-shuffled pack of 52 cards. If X denotes ‘ the number of aces drawn, then find the probability distribution of X.
Answer:
Let X denotes the number of aces in a sample of 4 cards drawn from a well shuffled pack of 52 cards.
So X can take values 0, 1,2, 3, 4.
P(X = 0) = probability of getting no ace
= \(\frac{{ }^{48} C_4}{{ }^{52} C_4}\)
P(X = 1) = probability of getting one ace and 3 other cards
= \(\frac{{ }^4 C_1 \times{ }^{48} C_3}{{ }^{52} C_4}\)
P(X = 2) – prob. of getting two aces and 2 other cards
= \(\frac{{ }^4 C_2 \times{ }^{48} C_2}{{ }^{52} C_4}\)
P(X = 3) = prob. of getting three aces and one other card
= \(\frac{{ }^4 C_3 \times{ }^{48} C_1}{{ }^{52} C_4}\)
P(X = 4) = probability of getting four aces
= \(\frac{{ }^4 C_4}{{ }^{52} C_4}\)
The probability distribution of random variable X is given as under:
